Quest for High-Temperature Superconductors: Difference between revisions
PeterMorales (talk | contribs) No edit summary |
PeterMorales (talk | contribs) No edit summary |
||
| Line 11: | Line 11: | ||
===BCS Theory=== | ===BCS Theory=== | ||
[[Image:BCS.jpg|thumb| | [[Image:BCS.jpg|thumb|150px|top|BCS theory group standing from left to right C B S]] | ||
[[Image:cooperpairs.jpg|thumb| | [[Image:cooperpairs.jpg|thumb|350px|right|illustrations of electrons traveling through a material in cooper-pairs]] | ||
[[Image:supercarriers.jpg|thumb| | [[Image:supercarriers.jpg|thumb|250px|right|Landau explained how superconducting pairs are lost at a certain temperature]] | ||
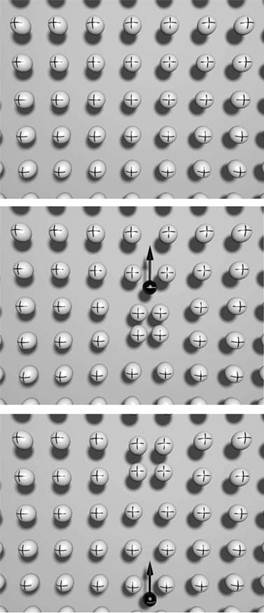
To explain super conductivity it was proposed that electrons in super conducting materials form pairs (cooper pairs). These pairs were shown by Cooper to be energetically favorable; it was energetically favorable to have an attraction between electron pairs. The energy required to break these pairs explained the energy gaps in superconductors and their dissimilarity to metals. However, cooper pairs alone did not solve the issue of superconductivity. That task remained to Bob Schrieffer, a graduate student working with Cooper under the supervision of Bardeem (the B in BCS and co-father of the transistor). Schrieffer attempted to find a wave function describing electrons in a superconducting material. For Cooper Pairs, Cooper had only considered one pair of electrons at a time and treated the rest of the 10^23 fermions as immobile, Schrieffer realized, while traveling by subway, that in order to represent the wave function properly, he had to consider all the electrons acting in harmony. In other words, the wave function would take into account the density of electrons and ignore the actual number discrete inside a material. This would lead to the BCS wave function. Superconductivity could now be described as an emergent property, similar to magnetism; a ‘macro’ quantum state due to a fixed phase of the electrons. In a superconductor, it was believed that all the individual electron’s wave functions were in phase. Also, Landau’s work on symmetry breaking, allowed for an explanation of how superconductivity undergoes a sudden transition from superconductor to non-superconductor. It seemed for the very first time that Superconductivity was explained. Unfortunately, BCS theory seemed to predict a theoretical maximum temperature near 30 degrees Kelvin. It seemed for now that Onnes’ dream of high temperature superconducting materials was impossible. | To explain super conductivity it was proposed that electrons in super conducting materials form pairs (cooper pairs). These pairs were shown by Cooper to be energetically favorable; it was energetically favorable to have an attraction between electron pairs. The energy required to break these pairs explained the energy gaps in superconductors and their dissimilarity to metals. However, cooper pairs alone did not solve the issue of superconductivity. That task remained to Bob Schrieffer, a graduate student working with Cooper under the supervision of Bardeem (the B in BCS and co-father of the transistor). Schrieffer attempted to find a wave function describing electrons in a superconducting material. For Cooper Pairs, Cooper had only considered one pair of electrons at a time and treated the rest of the 10^23 fermions as immobile, Schrieffer realized, while traveling by subway, that in order to represent the wave function properly, he had to consider all the electrons acting in harmony. In other words, the wave function would take into account the density of electrons and ignore the actual number discrete inside a material. This would lead to the BCS wave function. Superconductivity could now be described as an emergent property, similar to magnetism; a ‘macro’ quantum state due to a fixed phase of the electrons. In a superconductor, it was believed that all the individual electron’s wave functions were in phase. Also, Landau’s work on symmetry breaking, allowed for an explanation of how superconductivity undergoes a sudden transition from superconductor to non-superconductor. It seemed for the very first time that Superconductivity was explained. Unfortunately, BCS theory seemed to predict a theoretical maximum temperature near 30 degrees Kelvin. It seemed for now that Onnes’ dream of high temperature superconducting materials was impossible. | ||
Revision as of 09:04, 21 April 2011
The Quest Begins
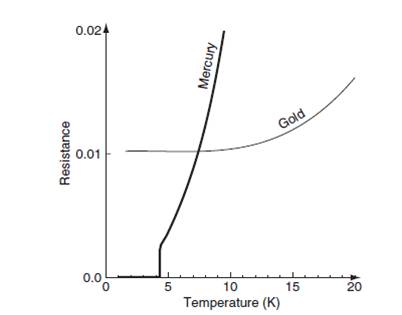
What originally began as a quest to reach absolute zero and solidify all the elemental gasses, eventually led to the discovery of Superconductivity in 1911. Earlier in the century, the discovery of chlorine had sparked a race amongst chemists to liquefy the elemental gasses. After the development of production techniques for liquid helium, new research investigations could begin on properties of materials at the low temperature scale. Kamerlingh Onnes, one of the world’s premiere low temperature experimentalists at the time, sought to solve the question of ‘what happens to the resistivity of metals at low temperatures? Several predictions had been made at the time by some extraordinary theorists. Upon testing the resistivity of Mercury, Onnes quickly discovered that at a critical temperature, the resistivity of the element completely vanished. Initially, Onnes was skeptical of the data, further investigation was needed. Onnes knew that a material with zero resistance would have a wide range of applications. After confirming his results, Onnes imagined creating large superconducting magnets from the material. Unfortunately, he soon discovered that applying a magnetic field to the material quickly destroyed the property of superconductivity. Before his death in 1926, Onnes correctly predicted the growth of low temperature physics laboratories, superconducting magnets, and other advancements in solid state physics. In addition to his tremendous legacy, Onnes left a challenge to future generations: To create superconducting materials that could withstand higher temperatures and carry stronger currents; higher temperatures, being the key to lowering cooling costs and increasing practicality.
Attempting to Explain Superconductivity
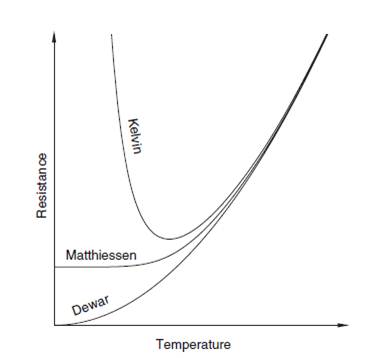
Since its discovery, several theories have been proposed to explain superconductivity. Yet to this day, there is no universally accepted theory. Superconductivity has baffled some of the greatest minds and lay waste to some of the most well-reasoned theoretical models in Physics. Originally, it was thought that because mercury was mostly free of impurities that it was imperfections in a material that caused resistance. This was due to the scattering of electrons traveling in the sample. This was proven incorrect by Onnes; adding impurities to mercury did not raise its resistance when below the critical temperature. It wasn’t until 1956 that theoretical work was able to produce a seeming sound model for superconductivity. BCS theory seemed to have finally explained the phenomena. However, in the 1980s, new superconducting materials were discovered that exceeded the limit proposed by BCS theory. Nevertheless, the concepts proposed in BCS theory still provide the modern framework for understanding superconductivity.
BCS Theory
To explain super conductivity it was proposed that electrons in super conducting materials form pairs (cooper pairs). These pairs were shown by Cooper to be energetically favorable; it was energetically favorable to have an attraction between electron pairs. The energy required to break these pairs explained the energy gaps in superconductors and their dissimilarity to metals. However, cooper pairs alone did not solve the issue of superconductivity. That task remained to Bob Schrieffer, a graduate student working with Cooper under the supervision of Bardeem (the B in BCS and co-father of the transistor). Schrieffer attempted to find a wave function describing electrons in a superconducting material. For Cooper Pairs, Cooper had only considered one pair of electrons at a time and treated the rest of the 10^23 fermions as immobile, Schrieffer realized, while traveling by subway, that in order to represent the wave function properly, he had to consider all the electrons acting in harmony. In other words, the wave function would take into account the density of electrons and ignore the actual number discrete inside a material. This would lead to the BCS wave function. Superconductivity could now be described as an emergent property, similar to magnetism; a ‘macro’ quantum state due to a fixed phase of the electrons. In a superconductor, it was believed that all the individual electron’s wave functions were in phase. Also, Landau’s work on symmetry breaking, allowed for an explanation of how superconductivity undergoes a sudden transition from superconductor to non-superconductor. It seemed for the very first time that Superconductivity was explained. Unfortunately, BCS theory seemed to predict a theoretical maximum temperature near 30 degrees Kelvin. It seemed for now that Onnes’ dream of high temperature superconducting materials was impossible.