Solution to Set 5

I have no idea what I'm doing - KimberlyWynne 03:11, 2 March 2009 (EST)

Diatomic harmonic chain

Problem 1

I found this site somewhat helpful and explanatory:

http://newton.ex.ac.uk/teaching/resources/rjh/phy2009/PHY2009handout13.pdf

Given

• a chain of atoms
• with alternating masses Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle m_1\;} and Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle m_2\;}
• connected with elastic springs with constant Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle K\;}
• moving only in the x-direction

Derive the dispersion relation $\omega ^{\alpha }(k)\;$ for this chain

Equations of motion

$m_{1}{\ddot {u}}_{n}=-k_{1}[2u_{n}-v_{n}-v_{n-1}]\;$

$m_{2}{\ddot {v}}_{n}=-k_{2}[2v_{n}-u_{n}-u_{n+1}]\;$

$u_{n}=u\mathrm {exp} (i(kna-\omega t))\;$

$v_{n}=v\mathrm {exp} (i(kna-\omega t))\;$

$-\omega ^{2}mu\mathrm {exp} (i(kna-\omega t))=k(v\mathrm {exp} (i(kna-\omega t))+v\mathrm {exp} (i(k(n-1)a-\omega t))-2u\mathrm {exp} (i(kna-\omega t)))\;$

$-\omega ^{2}m_{1}u=k(v+v\mathrm {exp} (-ika))-2ku\;$

$-\omega ^{2}m_{2}v=k(u\mathrm {exp} (ika)+u)-2kv\;$

Set determinant to 0

$\omega ^{2}=k({\frac {m_{1}+m_{2}}{m_{1}m_{2}}})\pm k{\sqrt {({\frac {m_{1}+m_{2}}{m_{1}m_{2}}})^{2}-{\frac {4}{m_{1}m_{2}}}sin^{2}({\frac {ka}{2}})]}}\;$

Step 1:

${\begin{vmatrix}2k-m_{1}\omega ^{2}&-C[1-\mathrm {exp} (-ika)]\\-C[1+\mathrm {exp} (-ika)]&2k-m_{2}\omega ^{2}\end{vmatrix}}=0$

Step 2:

${\begin{vmatrix}2k-m_{1}\omega ^{2}&-1\\-k&2k-m_{2}\omega ^{2}\end{vmatrix}}=0\;$

Step 3:

$(2k-m_{1}\omega ^{2})(2k-m_{2}\omega ^{2})-k(1)(-k)=0\;$

Problem 2

Determine the speed of sound for this chain. What is the lowest frequency of long-wavelength sound corresponding to the optical branch?

$\omega _{\alpha }(k)\approx C_{\alpha }k\;$

where

• $\omega _{\alpha }\;$ = frequency
• $C_{\alpha }\;$ = speed of sound
• $k\;$ = ???

Problem 3

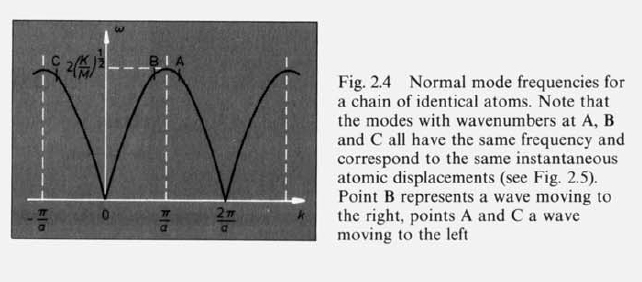
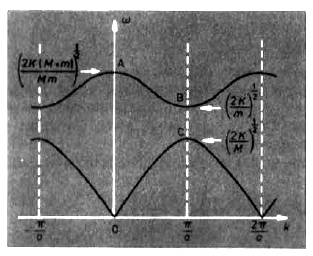
Sketch the motion of the atoms corresponding to the edge of the Brillouin zone, both for the optical and the acoustic branch.

• Acoustic Branch: lower branch
• Optical Branch: upper branch, as $k\rightarrow 0$ on this branch the vibrations of the 2 types of atom are in antiphase and the resulting charge oscillation in an ionic craystal give a strong coupling to electromagnetic waves at the frequency of point A.

Problem 4

Determine the Debye temperature for this system, and determine the form of the specific heat Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle C_V (T)} in the limits of high and low temperatures.

Debye Temperature Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle T_D \;}

The Debye temperature, aka the effective sonic velocity, is a measure of the hardness of the crystal

From our class lectures:

Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle k_B T_D = \hbar \omega_D = \hbar c k_D \;}

From Wikipedia:

Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle T_D\ \stackrel{\mathrm{def}}{=}\ {hc_sR\over2Lk} = {hc_s\over2Lk}\sqrt[3]{6N\over\pi} = {hc_s\over2k}\sqrt[3]{{6\over\pi}{N\over V}}}

Specific Heat Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle C_V \;}

Low Temperature Limit

Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \frac{C_V}{Nk} \sim {12\pi^4\over5} \left({T\over T_D}\right)^3}

High Temperature Limit

Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle T_{high} >> \frac{\hbar\omega}{k_B} \;}

Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \frac{\hbar\omega}{k_B T} << 1 \;}

Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle e^{\frac{\hbar\omega}{k_B T}} \approx 1 + \frac{\hbar\omega}{k_B T} + (\frac{\hbar\omega}{k_B T})^2 \;}

Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \frac{C_V}{Nk} \sim 3\, }

Net Result (Classical Limit)

Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle C_V \approx k_B \;}

Problem 5

Consider low temperatures (Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle T \ll T_D\;} ) and determine the wavelength of the most abundant phonons Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \lambda_{max}}

Given

• Bose-Einstein Distribution Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle f(E)=\frac{1}{e^{E / k_B T}-1} \;}
  • Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle f(E) \;} = probability that a particle will have energy E
  • Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle k_B \;} = Boltzmann constant
  • Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle T \;} = Temperature

• Planck's Radiation Formula Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle E = \frac{h \omega}{e^{h \omega / k_B T} - 1} \;}
  • Density by frequency: Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \rho (\omega) = \frac{dn_s}{d\omega} = \frac{8 \pi}{c^3} \omega^{2} \;}
  • Density by wavelength: Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \rho (\lambda) = \frac{dn_s}{d\lambda} = \frac{8 \pi}{\lambda^{4}} \;}

• Wien's law Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \lambda_{\mathrm{max}} = \frac{b}{T} \;}
  • Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \lambda_max \;} is the peak wavelength in meters,
  • Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle T \;} = temperature of the blackbody in Kelvin
  • Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle b \;} = Wien's displacement constant