Phy5645/Double pinhole experiment: Difference between revisions
No edit summary |
No edit summary |
||
| Line 10: | Line 10: | ||
[[Image:Double_pinhole_1.JPG]] | [[Image:Double_pinhole_1.JPG]] | ||
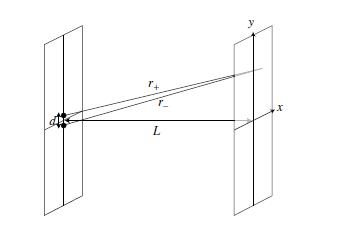
Suppose you send in an electron along the z axis on a screen at <math>z = 0</math> with two pinholes at <math>x = 0, y = \pm d/2</math>. On a point <math>(x,y)</math> on another screen at <math>z = L>>d, \lambda | Suppose you send in an electron along the z axis on a screen at <math>z = 0\!</math> with two pinholes at <math>x = 0, y = \pm d/2\!</math>. On a point <math>(x,y)\!</math> on another screen at <math>z = L>>d, \lambda\!</math>, the distance from each pinhole is given by <math> r_{\pm}=\sqrt{x^{2}+(y\mp d/2)^{2}+L^{2}}\!</math>. The spherical wave from each pinhole is added on the screen and hence the wave function is | ||
:<math>\psi(x,y)=\frac{e^{ikr_{+}}}{r_{+}}+\frac{e^{ikr_{-}}}{r_{-}}</math>, | :<math>\psi(x,y)=\frac{e^{ikr_{+}}}{r_{+}}+\frac{e^{ikr_{-}}}{r_{-}}</math>, | ||
| Line 37: | Line 37: | ||
:<math>|\psi_{+}(x,y)|^{2}+|\psi_{-}(x,y)|^{2}</math> | :<math>|\psi_{+}(x,y)|^{2}+|\psi_{-}(x,y)|^{2}\!</math> | ||
instead. Plot this function on y-axis, and also show the contour plot, to compare its pattern to the case when you do not place a counter. What is the difference from the case without the counter? | |||
-------- | |||
'''Answer:''' | |||
(a) As directed, we assume that the denominators are approximately the same between two waves. This is justified because the corrections are only of the order of <math>d/l\!</math>, and we are interested in the case where <math>d<<L\!</math>. We require that the numerators have the same phase, namely <math>kr_{+}-kr_{-}=2\pi n\!</math>. We expand the LHS with respect to <math>d\!</math>, | |||
:<math> | |||
\begin{align} | |||
k(r_{+}-r_{-})&\approx k\left\{\left(\sqrt{L^{2}+x^{2}+y^{2}}+\frac{yd}{2\sqrt{L^{2}+x^{2}+y^{2}}}\right) \right. \\ | |||
&-\left.\left(\sqrt{L^{2}+x^{2}+y^{2}}-\frac{yd}{2\sqrt{L^{2}+x^{2}+y^{2}}}\right)\right\}\\ | |||
&=k\frac{dy}{\sqrt{L^{2}+x^{2}+y^{2}}} | |||
\end{align} | |||
</math> | |||
Therefore,<math>k\frac{dy}{\sqrt{L^{2}+x^{2}+y^{2}}}=2\pi n\!</math> | |||
and hence | |||
:<math>\frac{y}{\sqrt{L^{2}+x^{2}+y^{2}}}=n\frac{\lambda}{d}\!</math> | |||
(b)Let us choose the unit where ''k'' = 1. Then we pick ''d'' = 20, ''L'' = 1000. Here is the interference pattern. First along the ''y''-axis (''x'' = 0): | |||
Revision as of 22:58, 5 December 2009
Submitted by team 1
Question: Double Pinhole Experiment
Besides Stern-Gerlach experiment, double slit experiment is another one that demonstrates how different quantum mechanics is from the classic counterpart. To avoid mathematical complications with Bessel function, we discuss two pinholes rather than slits.
Suppose you send in an electron along the z axis on a screen at Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle z = 0\!} with two pinholes at Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle x = 0, y = \pm d/2\!} . On a point Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle (x,y)\!} on another screen at Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle z = L>>d, \lambda\!} , the distance from each pinhole is given by Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle r_{\pm}=\sqrt{x^{2}+(y\mp d/2)^{2}+L^{2}}\!} . The spherical wave from each pinhole is added on the screen and hence the wave function is
- Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \psi(x,y)=\frac{e^{ikr_{+}}}{r_{+}}+\frac{e^{ikr_{-}}}{r_{-}}} ,
where Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle k = 2\pi /\lambda\!} . Answer the following questions.
(a) Considering just the exponential factors, show that the constructive interference appears approximately at
- Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \frac{y}{r}=n\frac{\lambda}{d}}
where Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle r=\sqrt{x^{2}+y^{2}+L^{2}}} .
(b) Make a plot of the intensity Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle |\psi(0,y)|^{2}\!} as a function of Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle y\!} , by choosing Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle k=1, d =20\!} , and Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle L=1000 \!} . The intensity Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle |\psi(0,y)|^{2}\!} is interpreted as the probability distribution for the electron to be detected on the screen, after repeating the same experiment many many times.
(c) Make a contour plot of the intensity Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle |\psi(x,y)|^{2}\!} as afunction of x and y, for the same parameters.
(d) If you place a counter at both pinholes to see if the electron has passed one of them, all of a sudden the wave function "collapses". If the electron is observed to pass through the pinhole at Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle y=+d/2\!} , the wave function becomes
- Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \psi_{+}(x,y)=\frac{e^{ikr_{+}}}{r_{+}}} .
If it is observed to pass through that at Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle y=-d/2\!} , the wave function becomes
- Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \psi_{-}(x,y)=\frac{e^{ikr_{-}}}{r_{-}}} .
After repeating this experiment many times with 50:50 probability for each the pinholes, the probability on the screen will be given by
- Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle |\psi_{+}(x,y)|^{2}+|\psi_{-}(x,y)|^{2}\!}
instead. Plot this function on y-axis, and also show the contour plot, to compare its pattern to the case when you do not place a counter. What is the difference from the case without the counter?
Answer:
(a) As directed, we assume that the denominators are approximately the same between two waves. This is justified because the corrections are only of the order of Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle d/l\!} , and we are interested in the case where Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle d<<L\!} . We require that the numerators have the same phase, namely Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle kr_{+}-kr_{-}=2\pi n\!} . We expand the LHS with respect to Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle d\!} ,
- Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \begin{align} k(r_{+}-r_{-})&\approx k\left\{\left(\sqrt{L^{2}+x^{2}+y^{2}}+\frac{yd}{2\sqrt{L^{2}+x^{2}+y^{2}}}\right) \right. \\ &-\left.\left(\sqrt{L^{2}+x^{2}+y^{2}}-\frac{yd}{2\sqrt{L^{2}+x^{2}+y^{2}}}\right)\right\}\\ &=k\frac{dy}{\sqrt{L^{2}+x^{2}+y^{2}}} \end{align} }
Therefore,Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle k\frac{dy}{\sqrt{L^{2}+x^{2}+y^{2}}}=2\pi n\!}
and hence
- Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \frac{y}{\sqrt{L^{2}+x^{2}+y^{2}}}=n\frac{\lambda}{d}\!}
(b)Let us choose the unit where k = 1. Then we pick d = 20, L = 1000. Here is the interference pattern. First along the y-axis (x = 0):