Phy5645/Double pinhole experiment: Difference between revisions
No edit summary |
No edit summary |
||
| Line 10: | Line 10: | ||
[[Image:Double_pinhole_1.JPG]] | [[Image:Double_pinhole_1.JPG]] | ||
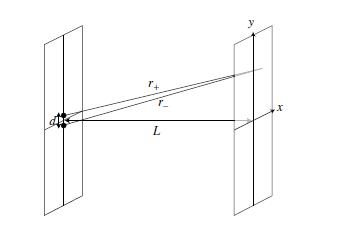
Suppose that a beam of electrons, traveling along the <math>z</math> axis, hits a screen at <math>z = 0 | Suppose that a beam of electrons, traveling along the <math>z</math> axis, hits a screen at <math>z = 0</math> with two pinholes at <math>x = 0, y = \pm d/2</math>. For a point <math>(x,y)</math> on a second screen at <math>z = L>>d, \lambda</math>, the distance from each pinhole is given by <math> r_{\pm}=\sqrt{x^{2}+(y\mp d/2)^{2}+L^{2}}</math>. A spherical wave is emitted from each pinhole; the waves from each add, and the wave function at a given point on the second screen is | ||
:<math>\psi(x,y)=\frac{e^{ikr_{+}}}{r_{+}}+\frac{e^{ikr_{-}}}{r_{-}}</math> | :<math>\psi(x,y)=\frac{e^{ikr_{+}}}{r_{+}}+\frac{e^{ikr_{-}}}{r_{-}},</math> | ||
where <math> k = 2\pi /\lambda | where <math> k = 2\pi /\lambda .</math> | ||
(a) Considering just the exponential factors, show that the constructive interference appears approximately at | (a) Considering just the exponential factors, show that the constructive interference appears approximately at | ||
| Line 20: | Line 20: | ||
:<math> \frac{y}{r}=n\frac{\lambda}{d}</math> | :<math> \frac{y}{r}=n\frac{\lambda}{d}</math> | ||
where <math> r=\sqrt{x^{2}+y^{2}+L^{2}}</math> | where <math> r=\sqrt{x^{2}+y^{2}+L^{2}}.</math> | ||
(b) Make a plot of the intensity <math>|\psi(0,y)|^{2} | (b) Make a plot of the intensity <math>|\psi(0,y)|^{2}</math> as a function of <math>y</math>, by choosing <math>k=1, d =20</math>, and <math> L=1000. </math> The intensity <math>|\psi(0,y)|^{2}</math> is interpreted as the probability distribution for the electron to be detected on the screen, after repeating the same experiment many many times. | ||
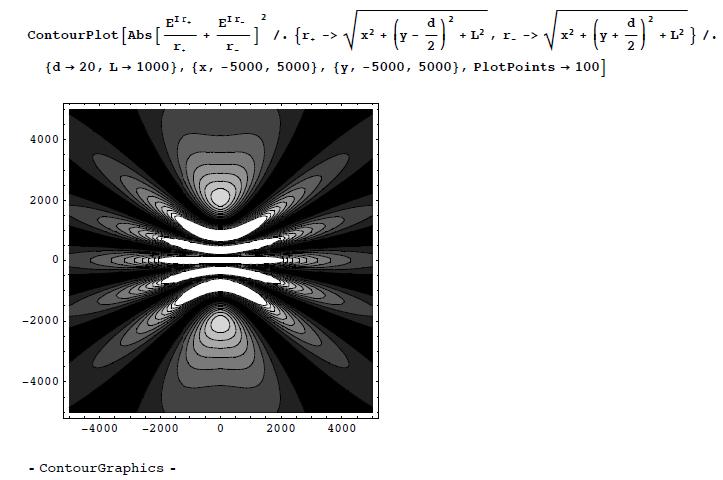
(c) Make a contour plot of the intensity <math>|\psi(x,y)|^{2} | (c) Make a contour plot of the intensity <math>|\psi(x,y)|^{2}</math> as a function of <math>x</math> and <math>y</math>, for the same parameters. | ||
(d) If you place a counter at both pinholes to see if the electron has passed one of them, all of a sudden the wave function "collapses". If the electron is observed to pass through the pinhole at <math>y=+d/2\!</math>, the wave function becomes | (d) If you place a counter at both pinholes to see if the electron has passed one of them, all of a sudden the wave function "collapses". If the electron is observed to pass through the pinhole at <math>y=+d/2\!</math>, the wave function becomes | ||
:<math>\psi_{+}(x,y)=\frac{e^{ikr_{+}}}{r_{+}}</math> | :<math>\psi_{+}(x,y)=\frac{e^{ikr_{+}}}{r_{+}}.</math> | ||
If it is observed to pass through that at <math>y=-d/2\!</math>, the wave function becomes | If it is observed to pass through that at <math>y=-d/2\!</math>, the wave function becomes | ||
:<math>\psi_{-}(x,y)=\frac{e^{ikr_{-}}}{r_{-}}</math> | :<math>\psi_{-}(x,y)=\frac{e^{ikr_{-}}}{r_{-}}.</math> | ||
After repeating this experiment many times with 50:50 probability for each the pinholes, the probability on the screen will be given by | After repeating this experiment many times with 50:50 probability for each the pinholes, the probability on the screen will be given by | ||
:<math>|\psi_{+}(x,y)|^{2}+|\psi_{-}(x,y)|^{2}\!</math> | :<math>|\psi_{+}(x,y)|^{2}+|\psi_{-}(x,y)|^{2}\!</math> | ||
instead. Plot this function on y-axis, and also show the contour plot, to compare its pattern to the case when you do not place a counter. What is the difference from the case without the counter? | instead. Plot this function on y-axis, and also show the contour plot, to compare its pattern to the case when you do not place a counter. What is the difference from the case without the counter? | ||
Revision as of 14:51, 16 April 2013
Submitted by team 1
Question: Double Pinhole Experiment
Besides the Stern-Gerlach experiment, the double slit experiment also demonstrates the difference between quantum mechanics and classical mechanics. Here, we will discuss a double pinhole experiment rather than a double slit experiment because the former is mathematically simpler and still embodies the basic physics that we wish to demonstrate.
Suppose that a beam of electrons, traveling along the axis, hits a screen at with two pinholes at . For a point on a second screen at , the distance from each pinhole is given by . A spherical wave is emitted from each pinhole; the waves from each add, and the wave function at a given point on the second screen is
where
(a) Considering just the exponential factors, show that the constructive interference appears approximately at
where
(b) Make a plot of the intensity as a function of , by choosing , and The intensity is interpreted as the probability distribution for the electron to be detected on the screen, after repeating the same experiment many many times.
(c) Make a contour plot of the intensity as a function of and , for the same parameters.
(d) If you place a counter at both pinholes to see if the electron has passed one of them, all of a sudden the wave function "collapses". If the electron is observed to pass through the pinhole at , the wave function becomes
If it is observed to pass through that at , the wave function becomes
After repeating this experiment many times with 50:50 probability for each the pinholes, the probability on the screen will be given by
instead. Plot this function on y-axis, and also show the contour plot, to compare its pattern to the case when you do not place a counter. What is the difference from the case without the counter?
Answer:
(a) As directed, we assume that the denominators are approximately the same between two waves. This is justified because the corrections are only of the order of , and we are interested in the case where . We require that the numerators have the same phase, namely . We expand the LHS with respect to ,
Therefore,
and hence
(b) Let us choose the unit where k = 1. Then we pick d = 20, L = 1000. Here is the interference pattern. First along the y-axis (x = 0):
(c) Now on the plane:
(d) For the same parameter as in (b), First along the y-axis (x = 0):
Now on the plane:
The main difference is the absence of the interference pattern.