PHZ3400 Midterm Two Solution

PHZ 3400 – Midterm Two Exam (with solutions) – April 10, 2009

Problem 1

Explain the concept of the "Thermodynamic Limit", and present the corresponding domain wall argument (derivation of a formula) to estimate the relaxation time as a function of system size, at T < Tc.

The partition function which applies in this case is Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle Z = \sum_{n}^{\infty}\varepsilon^{-\beta E_n}} where n = the number of particles.

However, symmetry breaking, including phase transitions, cannot occur for any finite system[1]. Thus systems must be sufficiently large, Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle n\rightarrow \infty} , in order to reach the thermodynamic limit and for spontaneous symmetry breaking to occur.

This illustrates the most energetically favorable configuration of the system. The green line represents the Domain Wall.

This illustrates the probability of states. Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \Delta E} is the energy of the Domain Wall

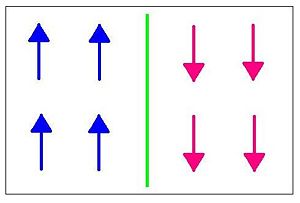
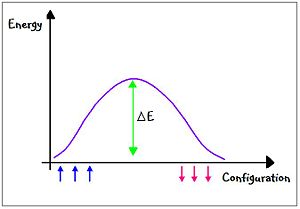
Suppose we start in a ferromagnetic system where all of the spins are up and we would like to make them spin down. This can be done by applying a domain wall to the system. The image to the left shows the most energetically favorable configuration of the system. This can be further described by the image to the right, which shows the probability of the system being in those states. Notice that the image shows the system is most likely to be found in a state where there is a mix of up and down spins. The domain wall will, by its nature, try to find the most energetically favorable state and it will thus lie where the energy of the probability of the states of the system is at a maximum.

Probability is represented mathematically as Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle P(E)\propto e^{-E/kT}} where E is the energy and T is the Temperature. When there is random motion, Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle P(E)\propto T} .

Since we are dealing with a domain wall, we can replace E with Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \Delta E} , which is also defined as Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \Delta E = \delta L} where Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \delta} is the surface tension Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \propto} J--the interaction between spins, and L is the size of the system.

With this substitution, we get Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle P(E) \propto e^{-E/kT} \propto \tau ^{-1} \propto e^{-AL^{d-1}/T}} .

d is the dimension, so for a three dimensional case we get Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle L^2} . The variable Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \tau} is the relaxation time, and the equation gives a relationship between the relaxation time and the size of the system.

The relaxation time and the size of the system have a direct correlation, as the size of the system increases the orelaxation time also increases, albeit at a greater rate. Thus if the size of the system is very large (macroscopic objects) then the relaxation time becomes larger than the age of the universe, and the system will stay in its current state.

Another interesting relation is between the relaxation time and temperature. As the temperature gets lower, the relaxation time gets larger. This fits in well with superconductors since they are only conducting at low temperatures. This means that as the semiconductors cool down the spins align and exhibit symmetry breaking.

[1] [http://prola.aps.org/abstract/PR/v87/i3/p404_1 C. N. Yang and T. D. Lee, Statistical Theory of Equations of State and Phase Transitions. I. Theory of Condensation Phys. Rev. 87, 404 - 409 (1952)]

Problem 2

Sketch the magnetization of a ferromagnet as a function of temperature T, for (A) Zero external magnetic field and (B) Finite external magnetic field. How is the behavior around the Curie Temperature (Tc) affected by the field?

Ignore the temperature but observe the trend as the magnetization approaches 0 at the Tc. Image is from [www.irm.umn.edu/hg2m/hg2m_b/Image9.gif]

This image shows the results for zero external magnetic field(blue) overlayed with the results with an external magnetic field(teal). Image from Dr. Dobrosavljevic's notes [1]

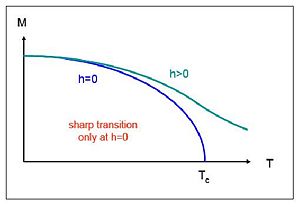
(A) For zero external magnetic field, the magnetization of a ferromagnet as a function of temperature T should go to the Curie Temperature (Tc). The Tc will be unique based on the ferromagnet.

Problem 3

Consider a solid with vibrations well described by the "Einstein Model", where a single vibrational frequency Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \omega_0} is considered. [Note: Phonons obey Bose-Einstein statistics.]

(A) What is the density of phonons with this frequency as a function of the temperature T? Sketch this function.

(B) What is the phonon density at very low temperatures?

(C) What is the phonon density at very high temperatures?

(D) Assuming that the scattering rate of electrons in such a solid is simply proportional to the density of phonons, determine the temperature dependence of resistivity at high temperatures.

Problem 4

Consider a neutron star of radius R = 10km, and mass M = 3x10³⁰ kg (approximately 1.5 solar masses). Given that the mass of a single neutron is m=1.7*10^-27 kg, that h-bar = 1x10^-34 m² kg/s, and that neutrons obey Fermi-Dirac Statistics, calculate:

a. The number density n=N/V of this star. N = total number of objects in volume V. Using this, we first can find the volume of the neutron star.

Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle V =\frac{4}{3} \pi R^3 \,}

b. Derive in detail the relation between Fermi energy and the number density.

Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle E_f = \frac{\hbar^2 \pi^2}{2 m L^2} n^2 \,} .

For a 3-D object, in this case a sphere,

Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle N =2\times\frac{1}{8}\times\frac{4}{3} \pi n_f^3 \,}

where the factor 2 comes from the fact that there are 2 spin states, 1/8 comes from the fact that only 1/8 of the sphere is positive, and Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \frac{4}{3} \pi n_f^3 \,} comes from the volume of the sphere with radius Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle n_f}

Solving for Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle n_f} and then substituting into Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle E_f = \frac{\hbar^2 \pi^2}{2 m L^2} n^2 \,} yields

Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle n_f=\left(\frac{3 N}{\pi}\right)^{1/3} }

and then finally

Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle E_f =\frac{\hbar^2 \pi^2}{2m L^2} \left( \frac{3 N}{\pi} \right)^{2/3}}

In order to get this in terms of number density, we substitute L² with V^2/3.