Motion in a Periodic Potential

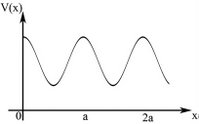
An example of a periodic potential is given in Figure 1 which consists of a series of continuous repeating form of potentials. In other words, the potential is translational symmetric over a certain period (in Figure 1 it is over period of Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle a\!} ).

Figure 1.

Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle V(x)=V(x + a) \!}

In this case Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle a \!} is the period.

The Hamiltonian of system under periodic potential commutes with the Translation Operator defined as:

Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \hat T_a\psi(x)=\psi(x+a)\!}

This means that there is a simultaneous eigenstate of the Hamiltonian and the Translation Operator. The eigenfunction to the Schrödinger equation,

Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \left(-\frac{\hbar^2}{2m}\frac{\partial^2}{\partial x^2}+V(x)\right)\psi(x)=E\psi(x)}

has the form of the following,

Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \psi(x)=e^{ikx}u_k(x)\!}

where

Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle u_k(x+a)=u_k(x)\!}

This result is also known as the Bloch Theorem.

Also, by operating the Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \hat T_a\!} operator on the wavefunction (also known as the Bloch wave), it can be seen that this waveform is also an eigenfunction of the Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \hat T_a\!} operator, as shown in the following,

Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \begin{align} \hat T_a\psi(x)&=\hat T_a \left(e^{ikx}u_k(x)\right)\\ &=\left(e^{ik(x+a)}u_k(x+a)\right)\\ &=e^{ika}\left(e^{ikx}u_k(x)\right)\\ &=e^{ika}\psi(x) \end{align}}

Using the same argument, it is clear that,

Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle (\hat T_a)^n\psi(x)=e^{ikna}\psi(x)}

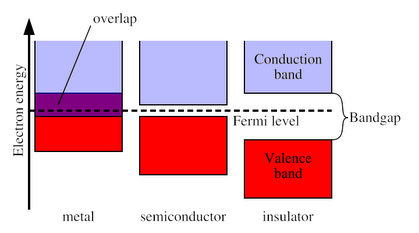
Also, note that if Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle k \!} is complex, then after multiple Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \hat T_a\!} operations, the exponential will "blow-up". Thus, Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle k \!} has to be real. Applying the Bloch Theorem in solving Schrödinger Equation with known periodic potential will reveal interesting and important results such as a band gap opening in the Energy vs Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle k \!} spectrum. For materials with weak electron-electron interaction, given the Fermi energy of the system, one can then deduce whether such a system is metallic, semiconducting, or insulating.

Figure 2. Energy band illustration showing the condition for metal, semiconductor, and insulator.

Consider for example the periodic potential and the resulting Schrödinger equation,

Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle V(x)=V_0\sum_{n=-\infty}^{\infty}\delta(x-na)}

Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \left(-\frac{\hbar^2}{2m}\frac{\partial^2}{\partial x^2}+V_0\sum_{n=-\infty}^{\infty}\delta(x-na)\right)\psi(x)=E\psi(x)}

Focusing the attention for case when Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle 0 < x < a \!} , the solution to the Schrödinger equation is of the form:

Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle E=-\frac{\hbar^2q^2}{2m}}

Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \begin{align} \psi(x) &= Ae^{iqx}+Be^{-iqx} \\ &= e^{ikx}\left(Ae^{i(q-k)x}+Be^{-i(q+k)x}\right) \\ &= e^{ikx}u_k(x) \end{align} }

From periodicity and continuity with Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \epsilon \rightarrow 0 } ,

Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle u_k(0 - \epsilon)=u_k(0 + \epsilon) = u_k(a + \epsilon)\!}

Thus, the wavefunction from Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle x < 0 \!} (left) and Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle x > 0 \! } (right) can be written as:

Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \psi_r=e^{ikx}u_k(x)=e^{ikx}(Ae^{i(q-k)x}+Be^{-i(q+k)x})\!}

Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \psi_r=e^{ikx}u_k(x+a)=e^{ikx}(Ae^{i(q-k)(x+a)}+Be^{-i(q+k)(x+a)})\!}

When the continuity requirement at x = 0 is also being imposed, the following relation is found:

Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \psi_l(0)=\psi_r(0)\!}

Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle A+B=Ae^{i(q-k)a}+Be^{-i(q+k)a}\!} (1)

From differentiability and periodicity, the Schrödinger equation can be solved as the following:

Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \int_{-\epsilon}^\epsilon -\frac{\hbar^2}{2m}\frac{\partial^2 \psi(x)}{\partial x^2}dx+\int_{-\epsilon}^\epsilon V_0\sum_{n=-\infty}^{\infty}\delta(x-na)\psi(x)dx=\int_{-\epsilon}^\epsilon E \psi(x)dx}

where Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \epsilon\!} is small, approaching zero. In this case, the term on the right hand side can be taken to be 0. Thus,

Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle -\frac{\hbar^2}{2m}(\frac{\partial\psi_r(\epsilon)}{\partial x}-\frac{\partial\psi_l(-\epsilon)}{\partial x})=-V_0\psi_l(0)}

Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \lim_{\epsilon\to 0}(\frac{\partial\psi_r(\epsilon)}{\partial x}-\frac{\partial\psi_l(-\epsilon)}{\partial x})=\frac{2mV_0}{\hbar^2}\psi_l(0)}

where,

Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \lim_{\epsilon\to 0}\frac{\partial\psi_r(\epsilon)}{\partial x}=iq(A-B)}

Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \lim_{\epsilon\to 0}\frac{\partial\psi_l(-\epsilon)}{\partial x}=iq(Ae^{i(q-k)a}-Be^{-i(q+k)a})}

Evaluating further, the following condition is found:

Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle iq(A-B-Ae^{i(q-k)a}+Be^{-i(q+k)a})=\frac{2mV_0}{\hbar^2}(A+B)} (2)

By simultaneously solving equation (1) and (2), the relationship between q and k is found to be:

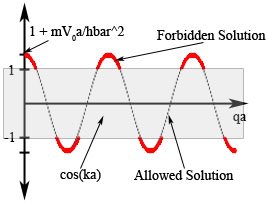
Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \cos(ka)=\cos(qa)+\frac{mV_0a}{\hbar^2}\frac{\sin(qa)}{qa}} (3)

Worked Problem on Periodic Delta Function Potentials: See Problem

Where q is the energy band of the system, and k is the accessible energy band. By noting that the maximum value of the LHS is < the maximum value of the RHS of the relation above, it is clearly seen that there are some energy level that are not accessible as shown in Figure 3.

Figure 3. Graph of Eq.(3). Wave is representing the LHS function, gray box representing the range of RHS function. Red lines is the forbidden solution, black line is the allowed solution.

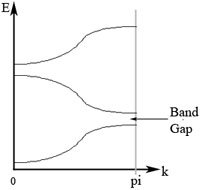
As k increases from 0 to , there will be many solutions. Focusing only to two of the allowed solutions, it is seen that as k increases, there will be two solutions (one solution gives increasing q value, the other solution gives decreasing q value). Using the fact that the energy of the system is , the dispersion relation (E vs. k) can be plotted as shown in Figure 4.

Figure 4. Energy vs k showing the existence of band gap for system of an electron under periodic potential.

Thus, in conjunction with Pauli exclusion principle, the single particle banspectrum such as the one we discussed here constitutes a simple description of band insulators and band metals.

If we take a more general case, where the potential is also periodic and always finite. The potential can be expressed as follows:

Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle V(x)=\begin{cases} 0, na<x<na+c;\\ V_0, na+c<x<(n+1)a. \end{cases} }

Further, we assume that the eigenfunction is:

Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \psi_r=e^{ikx}u_k(x)\! }

Then this problem is converted to seeking for Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle u_k(x)\!} in the region -b<x<c and connecting the wave function in different periods.

Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \left(-\frac{\hbar^2}{2m}\frac{\partial^2}{\partial x^2}\right)\psi(x)=E_k\psi(x), 0<x<c}

Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \left(-\frac{\hbar^2}{2m}\frac{\partial^2}{\partial x^2}+V_0\right)\psi(x)=E_k\psi(x), -b<x<0}

Further we assume that

Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle k_1^2=\frac{2mE_k}{\hbar^2}, k_2^2=\frac{2m(V_0-E_k)}{\hbar^2} }

Then we can get the equation for Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle u_k(x)\!} :

Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \frac{d^2u_k(x)}{x^2}+2ik\frac{du_(x)}{dx}+(k_1^2-k^2)u(x)=0, 0<x<c }

Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \frac{d^2u_k(x)}{x^2}+2ik\frac{du_(x)}{dx}-(k_2^2+k^2)u(x)=0, -b<x<0 }

Solve for Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle u_k(x) \!} , we get:

Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle u_k(x)=A_0e^{i(k_1-k)x}+B_0e^{-i(k_1+k)x}, 0<x<c }

Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle u_k(x)=C_0e^{(k_2-ik)x}+D_0e^{-(k_2+ik)x}, -b<x<o }

By the continuity of Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \psi(x) \!} and Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \frac{d\psi(x)}{dx}} at $x=0\!$:

$A_{0}+B_{0}=C_{0}+D_{0}\!$

$i(k_{1}-k)A_{0}-i(k_{1}+k)B_{0}=(k_{2}-ik)C_{0}-(k_{2}+ik)D_{0}\!$

By periodicity of $u_{k}(x)\!$, we get:

$u_{k}(x)=u_{k}(x+a)\!$

$C_{0}e^{(k_{2}-ik)x}+D_{0}e^{-(k_{2}+ik)x}=C_{1}e^{(k_{2}-ik)(x+a)}+D_{1}e^{-(k_{2}+ik)(x+a)}$

$C_{1}=C_{0}e^{-(k_{1}-ik)a}$

$D_{1}=D_{0}e^{(k_{2}+ik)a}$

By the continuity of $\psi (x)\!$ and ${\frac {d\psi (x)}{dx}}\!$ at $x=c\!$:

${\begin{aligned}A_{0}&e^{i(k_{1}-k)x}+B_{0}e^{-i(k_{2}+k)x}\\&=C_{1}e^{(k_{2}-ik)c}+D_{1}e^{-(k_{2}+ik)c}\\&=C_{0}e^{-(k_{2}-ik)b}+D_{0}e^{(k_{2}+ik)b}\end{aligned}}$

${\begin{aligned}i(k_{1}-k)&e^{i(k_{1}-k)c}A_{0}-i(k_{1}+k)e^{i(k_{1}+k)c}B_{0}\\=(k_{2}&-ik)e^{(k_{2}-ik)c}C_{1}-(k_{2}+ik)e^{-(k_{2}+ik)c}D_{1}\\=(k_{2}&-ik)e^{-(k_{2}-ik)b}C_{0}-(k_{2}+ik)e^{(k_{2}+ik)b}D_{0}\end{aligned}}$

Now, we get four linear equations of $A_{0},B_{0},C_{0}\!$ and $D_{0}\!$, to get the nontrivial solution, we have to make the determinant be zero:

${\frac {k_{2}^{2}-k_{1}^{2}}{2k_{1}k_{2}}}\sinh(k_{2}b)\sin(k_{1}c)+\cosh(k_{2}b)\cos(k_{1}c)=\cos(ka)$

This is the equation that the energy follows, where $k_{1}^{2}={\frac {2mE_{k}}{\hbar ^{2}}}\!$ and $k_{2}^{2}={\frac {2m(V_{0}-E_{k})}{\hbar ^{2}}}\!$.

If we take the limit $V_{0}\rightarrow 0\!$ and $b\rightarrow 0\!$ and maintain $V_{0}b\!$ finite, then we can obtain:

$k_{2}={\sqrt {{\frac {2m}{\hbar ^{2}}}(V_{0}-E_{k})b^{2}}}\simeq {\sqrt {(V_{0}b){\frac {2mb}{\hbar ^{2}}}}}\ll 1$

$sinh(k_{2}b)\simeq k_{2}b$

$cosh(k_{2}b)\simeq 1$

Hence we get:

$\cos(ka)=\cos(k_{1}a)+{\frac {mV_{0}ab}{\hbar ^{2}}}{\frac {\sin(k_{1}a)}{k_{1}a}}$

This equation is the same as the equation we obtain on the condition of periodic delta potential. And we should be aware that $V_{0}b$ is finite and stand for the $V_{0}$ in the previous problem.