Homeworks 4

Homework 4 due on 09/28/2009

Problem 1

A spectroscopic, eclipsing binary system has an orbital period of 6 years, and the maximum radial velocities are 5 and 22 km/sec, respectively. The period between first contact and minimum light ($t_{b}$ − $t_{a}$) is 0.6 days, and the length of the primary minimum ($t_{c}$ − $t_{a}$) is 0.6 days. The apparent bolometric magnitudes at maximum, primary and secondary minimum at 5.40, 9.2 and 5.44 magnitudes, respectively.

Given

• P = 6 years
• v₁ = 5 km/sec = 5000 m/s
• v₂ = 22 km/sec = 22000 m/s
• t_b − t_a = 0.6 days
• t_c − t_a = 0.6 days

Bolometric Magnitudes

• U = 5.40
• B = 9.2
• V = 5.44
• m_bol = V + BC

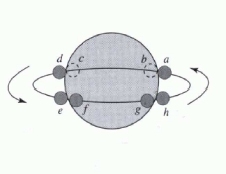
a) Describe in your own words, how you can derive the observables from the LCs given below.

LCs = light curves (Chapter 7.1)

For binaries that have orbital planes oriented approximately along the line of sight of the observer, one star may periodically pass in fron to fthe other, blocking the light of the eclipsed component (see Fig 7.2). Such a system is recognizable by regular variations in the amount of light received at the telescope. Not only do observations of these light curves reveal the presence of two stars, but the data can also provide information about relative effective temperatures and radii of each star based on the depths of the light curve minima and the lengths of the eclipses.

Assuming spherical orbits, determine b) the total mass of the system,

$M_{total}=m_{1}+m_{2}\;$

c) the individual masses,

Center of Mass

$m_{1}r_{1}=m_{2}r_{2}\;$

$m_{1}=m_{2}{\frac {r_{2}}{r_{1}}}$

$m_{2}={\frac {m_{1}+m_{2}}{1+{\tfrac {r_{2}}{r_{1}}}}}$

d) the individual radii, and

$d_{1}=\int _{a}^{b}v_{1}tdt=(5000m/s)(51840s)=2.592\times 10^{8}m$

$d_{2}=\int _{a}^{c}v_{2}tdt=(22000m/s)(51840s)=1.140\times 10^{9}m$

Circumference

$C_{1}=2\pi R_{1}=d_{1}$

$C_{2}=2\pi R_{2}=d_{2}$

Radius

$R_{1}={\frac {d_{1}}{2\pi }}=4.125\times 10^{7}m$

$R_{2}={\frac {d_{2}}{2\pi }}=1.814\times 10^{8}m$

e) the ratio of effective temperatures.

${\frac {T_{1}}{T_{2}}}={\frac {L_{1}/(4\pi \sigma R_{1}^{2})^{1/4}}{L_{2}/(4\pi \sigma R_{2}^{2})^{1/4}}}$

Problem 2

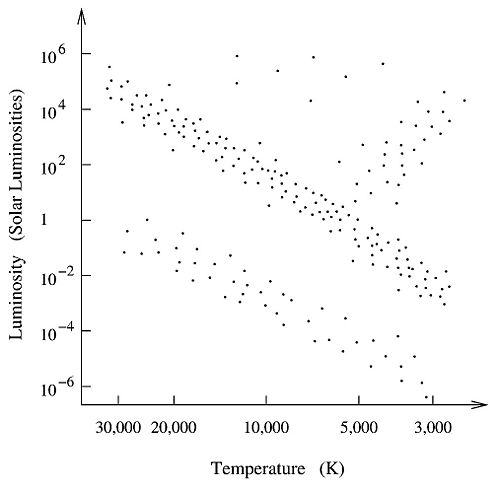
Sketch the H-R diagram indicating the location of main sequence stars, red giants, and white dwarfs. Label the axes with the correct temperatures and luminosities.

Problem 3

Two stars, A and B, have the same temperature, but their luminosities are 9.0 and 0.25 times the luminosity of the sun respectively. What is the ratio of the surface areas of the two stars? What is the ratio of their radii?

Problem 4

Problem 5

In first order, stellar spectra can be described by a black body (Planck function) of a certain temperature T based on the flux distribution in the optical wavelength range. Derive an expression for the frequency integrated flux as a function of T (show the steps), and provide an expression for the total luminosity of a star with Radius R. Estimate the total luminosity of the sun assuming a radius R of 6.96E10 cm and, based on its color, a temperature of 5400 K. Compare the result with the bolometric luminosity from literature.