Midterm Solution

Problem 1

Describe the difference between a first order and a second order phase transition. Give examples of each type. (10 points)

When determining the type of phase transition you must look at the change in the physical quantities of the material. If the change is sudden, a discontinuity in the first derivative of the free energy, then you have a first order phase transition. Examples of this would be water going from a liquid state to a gaseous state. A second order phase transition is continuous in the first derivative of the free energy and displays discontinuity only in the second derivative. With this fact, second order phase transitions will experience spontaneous symmetry breaking. Examples of a second order phase transition includes ferromagnetism, antiferromagnetism, and superconductivity.

Problem 2

Give some examples of spontaneous symmetry breaking. How does it depend on the system size? Explain how it can happen, in apparent violation of the ergodicity hypothesis of Boltzmann. According to Boltzmann, what determines the probability of some microscopic configuration (10 points)

Examples of spontaneous symmetry breaking are the same as those in second order phase transitions ferromagnetism, antiferromagnetism, and superconductivity. In order to have spontaneous symmetry breaking you must have an infinitely large system. If we have a finite system, spontaneous symmetry breaking could not occur. An example of spontaneous symmetry breaking as previously stated would be ferromagnetism. If we have a finite number of spins in our system then you will not be able to find a phase transition to the ferromagnetic state. With an infinite number of spins you will be in the thermodynamic limit and a ferromagnetic state will be able to be found. Boltzmann's ergodicity hypothesis states, in equilibrium each possible state of the system has the same energy and the same probability of existing. This means each possible state is equally populated. Viewing this hypothesis the system would transition over time visiting each possible state. The system does not necessarily violate the ergodicity hypothesis. The problem is the transition to each possible state can sometimes take much longer than our lifetimes or maybe even longer than the existence of the universe. We can determine this time as it is proportional to the probability. Looking at the probability of a microscopic configuration we get:

$P\thicksim e^{\sigma *L^{2}/T}$

Realistically these systems will not be microscopic, which will increase the L. It can then be seen why the system will take so long to transition as it increase exponentially with the system size.

Problem 3

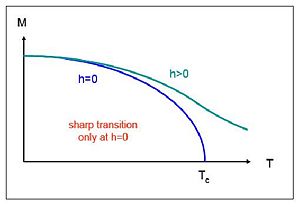
What determines the Curie temperature of a ferromagnet? How does it depend on the coordination number of the corresponding crystal lattice? Sketch the magnetization as a function of temperature in zero and in finite magnetic field. How does the field affect the transition? (10 points)

Their are two main components which determine the Curie Temperature of a ferromagnet. One is the coordination number of the material and the other is the magnetic exchange interaction. Given by the mean field theory, these two elements are proportional to each other when determining the Curie temperature. In our case the coordination number is the number of neighbors each magnetic spin has. The coordination number is determined by the type of crystal lattice. The higher the coordination number for each magnetic spin the more neighbors it has that can influence its spin direction. The magnetic exchange interaction is when two identical particles wave functions overlap and cause the spin expectation of each to change up or down.

The graph with an external magnetic field is sketched out in green and the graph without an external magnetic field is sketched in blue

Without external magnetic field

When looking at the graph of a present external magnetic field we can see the Curie Temperature is never reached, which is certainly a difference from the situation with an absent external magnetic field. Instead the graph will become asymptotic to zero.

Problem 4

The mean-field equation of state for a ferromagnet is given by the expression

$m=\tanh \left(\beta Jzm+\beta h\right)$.

Consider the system at high temperature, where nonzero magnetization is found only in presence of an external magnetic field. Assume that both the field and the magnetization are small, and determine (linearizing the RHS in m and h) the magnetization as a function of temperature, in presence of a weak field. Calculate the magnetic susceptibility and sketch its inverse as a function of T. Where does it diverge? (30 points)

The first step we need to take is to linearize the RHS of the equation. We can do this by considering only the first term of the taylor series expansion. The expansion of tanh(x) is the following:

${tanh(x)=x-{\frac {1}{3}}x^{3}+{\frac {2}{15}}x^{5}+...}$

By defining our x and only considering the first term of the expansion we are left with:

$m=\left(\beta Jzm+\beta h\right)$

Solving for m we get the following:

$m-\beta Jzm=\beta h\$

$m(1-\beta Jz)=\beta h\$

${m={\frac {\beta h}{1-\beta Jz}}}$

Tc and T are defined as the following:

$\ T_{c}=Jz\$

$\ T={\frac {1}{k_{B}B}}\$

Substituting in Tc and T we get:

$m={\cfrac {h}{Tk_{B}(1-{\cfrac {T_{c}}{Tk_{B}}})}}$

${m={\frac {h}{Tk_{B}-T_{c}}}}$

Setting kB equal to one we get the magnetitzation as:

${m\approx {\frac {h}{T-T_{c}}}}$

We can define the magnetic susceptibility as the following:

$\chi ={\frac {\partial M}{\partial H}}$

The the derivative of m with respect to h we get:

$\chi ={(T-T_{c})}^{-1}$

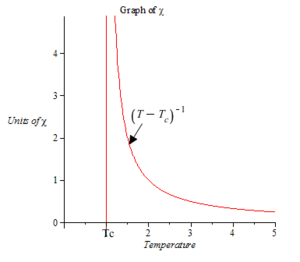
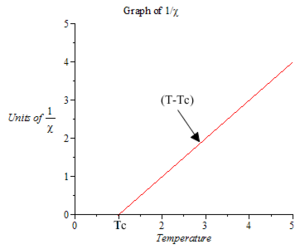
Finding the inverse we get:

${\frac {1}{\chi }}={(T-T_{c})}$

Graphing both of these we have the following:

Not the Inverse of Chi

Inverse of Chi

We can see from both of these graphs that that the magnetic susceptibility diverges as it approaches the critical temperature (Tc).

Problem 5

What is the physical principle behind the fact that atoms act as hard spheres, i.e. they have a “core” impenetrable to other atoms? (10 points)

Due to the Pauli Exclusion Principle all the core electrons of an atom cannot occupy the lowest energy state. Instead they must occupy different energy states. As a result this does not allow neighboring atom's core electrons to penetrate. This creates an impenetrable core and is the reason why we can have solid structures.

Problem 6

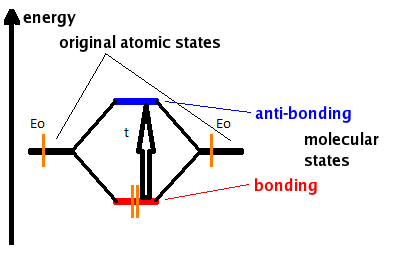
Consider a molecule consisting of two identical atoms. Assume that the ground state energy of each atom is $E_{o}$, and that the corresponding hopping element between the two atoms is $t(R)$. Calculate the energy difference between the bonding and the anti-bonding molecular state for this molecule. How do you expect it to depend on the inter-atomic distance R? What is the energy needed to break the molecule, i.e. the energy of the covalent bond that binds the atoms in the molecule? (30 points)

The first step we need to do is write down the Schrödinger Equation:

$\ {{\hat {H}}{\Psi _{E}}}={E{\Psi _{E}}}$

Where: $\ {\hat {H}}={\frac {\hbar }{2m}}\nabla ^{2}+V(r)$

Using Dirac Notation we can write the Schrödinger Equation as the following:

${\hat {H}}|\Psi \rangle =E|\Psi \rangle$

We know we can right the molecular wave function as a linear combination of the 2 atoms in the molecule.

$\ H[a_{1}|\Psi _{1}\rangle +a_{2}|\Psi _{2}\rangle ]=E[a_{1}|\Psi _{1}\rangle +a_{2}|\Psi _{2}\rangle ]$

We can get 2 equations by multiplying by Ψ1 and Ψ2

Noting that when we apply Ψ1 onto Ψ1(or any wave function onto itself) we will get 1. Also when we apply Ψ1 onto Ψ2 (or vise versa) we will get zero.

$\ \langle \Psi _{1}|\Psi _{1}\rangle =1$

$\langle \Psi _{1}|\Psi _{2}\rangle =0$

Multiplying through by Ψ1 we get:

$\ a_{1}\langle \Psi _{1}|H|\Psi _{1}\rangle +a_{2}\langle \Psi _{1}|H|\Psi _{2}\rangle =Ea_{1}$

We can then see we find our overlapping matrix element and our ground state energy left in the equation as:

$\langle \Psi _{1}|H|\Psi _{1}\rangle =\epsilon _{0}$

$\langle \Psi _{1}|H|\Psi _{2}\rangle =-t$

Writing out the simplified version of our first equation we are left with:

$\ a_{1}(\epsilon _{0})+a_{2}(-t)=Ea_{1}$

When we multiply through by Ψ2 we will get the following:

$\ a_{1}\langle \Psi _{2}|H|\Psi _{1}\rangle +a_{2}\langle \Psi _{2}|H|\Psi _{2}\rangle =Ea_{2}$

Simplifying our second equation we are left with:

$\ a_{1}(-t)+a_{2}(\epsilon _{0})=Ea_{2}$

We can now write this as a homogeneous set of linear equations:

${\begin{bmatrix}\epsilon _{0}&-t\\-t&\epsilon _{0}\\\end{bmatrix}}{\begin{bmatrix}a_{1}\\a_{2}\\\end{bmatrix}}=E{\begin{bmatrix}a_{1}\\a_{2}\\\end{bmatrix}}$

We can solve this by using Eigen value analysis. We know for an NxN matrix we will have N number of solutions or N possible values of the Eigenvalue E. To find the Eigenvalues we can use the following equation:

$\ det[{\hat {m}}-E{\hat {I}}]=0$

Where m is our matrix and I is the identity matrix.

Rewriting our equation we have the following:

${\begin{bmatrix}\epsilon _{0}-E&-t\\-t&\epsilon _{0}-E\\\end{bmatrix}}=0$

Solving the matrix by finding the determinant we are left with:

$\ (\epsilon _{0}-E)^{2}-t^{2}=0$

Simplifying the equation we find the energy to be:

$\ E=\epsilon _{0}\pm t(R)$

By looking at the time dependent solution of the Schroedinger Equation we can see that the energy is affected exponentially with respect to the inter-atomic distance R.

$\ |\Psi (t)\rangle =e^{-it{\frac {E}{R}}}|\Psi _{E}\rangle$

Taking a looking at a graph of the bonding and antibonding state we have:

The energy needed to break a covalent bond is the binding energy. This energy is the energy difference in the bound and unbound states. Looking at our diagram we can see that is the hopping element t(R).