PHZ3400-09 Modern Physics

“ Ah, the reason we all majored in physics in the first place... ”

Group 3 is the best

Modern Physics

Society of Physics Students

Atomic Structure
Physics	Relativity Quantum Mechanics Structure of Matter
Century	20th - 21st
Giants
Classical	J.J.Thompson Rutherford Bohr Rydberg Newton
Quantum^[1]	Einstein Heisenburg Fermi Pauli Planck de Broglie Schrodinger

Modern Physics (Greek: physis – φύσις meaning "nature") refers to the branches of physics developed from the discovery of the quantum nature of physics at the beginning of the 20th century. More generally, the term can refer to any branch of physics developed from this time period onward.

Modern Physics introduces the exciting developments of physics in the 20th Century (and some of the 21st). Well, anything that happened after PHY2049. We will see how the seeds of this great revolution were planted in the greatest triumph of the 19th century physics! And a revolution it was! There is no other way to describe the fascinating, surprising, and mind boggling discoveries of relativity, quantum mechanics, and the structure of matter. Some of the great scientists we will encounter along the way are Planck, Einstein, Curie, Rutherford, Bohr, Pauli, Dirac, Schrodinger, Heisenburg, Fermi, Geynman, Rydberg, and Schrieffer. Two of these giants of the 20th century physics, Dirac and Schrieffer, have served on the FSU faculty!

Introduction

Notes: Lecture
Lecturer: Dr. Vlad's Friend
Time: 12:20pm - 1:10pm
Dates: Wednesday, January 14, 2009 & Friday, January 16, 2009
Place: The Classroom Building, HCB 317, Florida State University

“ At the end of the 19th century, scientists had gotten quite a lot done. But there was no explanation for things that happened at the quantum level. What happened when things got very small and very fast? ”

Black Body Radiation

Intensity vs. Wavelength

One of the mysteries that led to a break through in modern physics, was that of thermal radiation. Thermal Radiation is electromagnetic radiation that is emitted by all objects merely because of their temperature. The classical wave picture of electromagnetic radiation failed to explain this phenomenon.

Early observations found that the total intensity radiated over all wavelengths increases as the temperature increases. Careful measurements found that the total intensity increases as the fourth power of the temperature:

Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle I = \sigma T^{4}\!}

This equation is known as Stefan's Law, where Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \sigma} is known as the Stefan-Boltzmann constant.

A second observation made was that the wavelength at which the radiancy reaches a maximum value decreases as the temperature is increased, in inverse proportion to the temperature. Careful observations determined that the proportionality constant, such that:

Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \lambda_{max} T = 2.898 x 10^{-3} m\cdot K\!}

This equation is known as Wien's displacement law.

Since the amount of radiation emitted by a solid object depends not only on the temperature, but also on other factors, such as the properties of the surface, physicists simplified the problem by considering an object whose surface is completely black, or a blackbody. Using this simplification, physicists were able to ignore any radiation being reflected from the surface of the object.

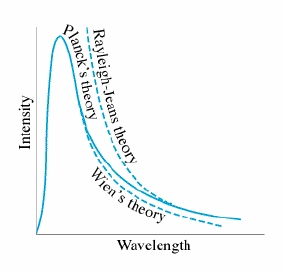
The closest that classical physics came to describing blackbody radiation was the Rayleigh-Jeans formula, which was based firmly on the classical theories of electromagnetism and thermodynamics.

Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle R(\lambda) = \frac{8\pi}{\lambda^4}kT\frac{c}{4}\!}

However, this was only able to predict radiance at high wavelengths compared against observations (as seen in the graph). The failure of the Rayleigh-Jeans formula (and thereby the failure of classical physics to explain blackbody radiation) is known as the ultraviolet catastrophe.

Planck's Hypothesis

Max Planck

The new physics that gave the correct interpretation of thermal radiancy was proposed by the German physicist Max Planck in 1900. Planck needed to formulate a new model to unite two conflicting mathematical expressions for blackbody radiation spectra: the Wein approximation, which is accurate at short wavelengths but gives too much radiance at long wavelengths, and the Rayleigh-Jeans law, which has the opposite problem. Planck's breakthrough came when he realized that he could treat matter as a collection of charged oscillators (today known to be atoms) and considering the different ways in which electromagnetic energy can be distributed over their various modes of oscillation. He found that when he suggested that an oscillating atom can absorb or re-emit energy only in discrete bundles, or quanta, his now-famous law emerged and fit the data quite well. If the energy of the quanta were proportional to the frequency of the radiation, then as the frequencies became large, the energy would similarly become large. Since no individual wave could contain more than Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle kT} (the mean total energy of an oscillating atom) of energy, then no standing wave could exist whose energy quantum was larger than Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle kT} . This effectively limited the high-frequency (short-wavelength) radiant intensity, and subsequently solved the ultraviolet catastrophe (which, contrary to popular belief, was not actually noticed until five years after Planck derived his law).

In Planck's theory, each oscillator can emit or absorb energy only in quantities that are integer multiples Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle n} of a certain basic quantity of energy Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \epsilon} ,

Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle E = n\epsilon\!}

The energy of each mode of oscillation is quantized. Radiation comes from electric oscillators. Furthermore, the energy of each of the quanta is determined by the frequency

Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \epsilon = h\nu\!}

where Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle h} is Planck's constant. Based on this assumption, Planck calculated the radiancy to be

Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle R(\lambda) = (\frac{c}{4})(\frac{8\pi}{\lambda^4})[(\frac{hc}{\lambda})\frac{1}{e^{\frac{hc}{\lambda kT}}-1}]\!}

Specific Heat of Solids

Specific heat is represented by C, where Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \frac{C_v}{N_A} = const.}

The specific heat of a substance is the rate of change of the energy with respect to temperature.

Dulong-Petit Law

In classical physics, the Dulong-Petit law, a chemical law proposed in 1819 by French physicists and chemists Pierre Louis Dulong and Alexis Thérèse Petit, states the expression for the specific heat capacity of a crystal due to its lattice vibrations.

Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle C_{v} = \frac{\partial \epsilon (T)}{\partial T} = 3nk_{B}}

The result is extremely simple; regardless of the nature of the crystal, the specific heat capacity (in joule per kelvin per kilogram) is equal to 3R/M, where R is the gas constant (measured in joule per kelvin per mole) and M is the molar mass (measured in kilogram per mole). In other words, the dimensionless heat capacity is equal to 3.

Despite its simplicity, Dulong-Petit law offers fairly good prediction for the specific heat capacity of solids with relatively simple crystal structure at high temperatures.

However, it fails in the low temperature region where C drops sharply and approaches zero. Classical physics fails and the quantum mechanical nature of the solid manifests itself. Thus, the Debye model takes the stage:

Debye model

The Debye model is a method developed by Peter Debye in 1912[1] for estimating the phonon contribution to the specific heat (heat capacity) in a solid. It treats the vibrations of the atomic lattice (heat) as phonons in a box, in contrast to the Einstein model, which treats the solid as many individual, non-interacting quantum harmonic oscillators. The Debye model correctly predicts the low temperature dependence of the heat capacity, which is proportional to Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle T^3} . In this model, phonons are the quantization of lattice vibrations. Just like the Einstein model, it also recovers the Dulong-Petit law at high temperatures. But due to simplifying assumptions, its accuracy suffers at intermediate temperatures.

Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \frac{C_V}{Nk} = 9 \left({T\over T_D}\right)^3\int_0^{T_D/T} {x^4 e^x\over\left(e^x-1\right)^2}\, dx\,.}

Photoelectric Effect

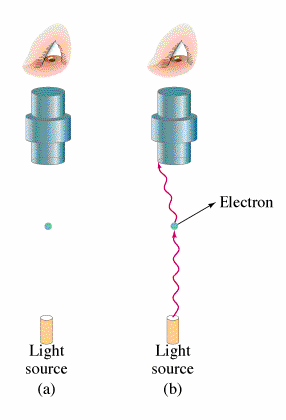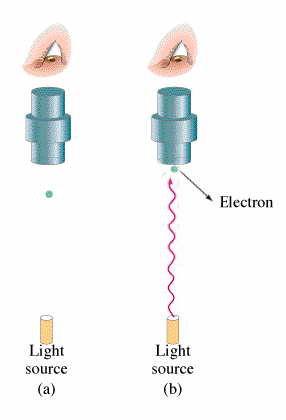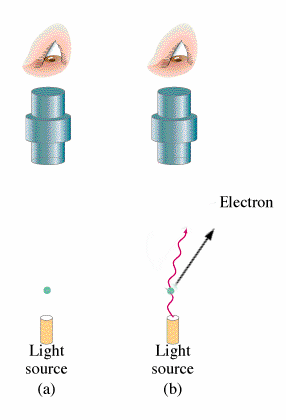
Einstein won the Nobel prize for the Photoelectric Effect but not for his more popular work on general relativity. The photoelectric effect does however have huge implications for modern physics and provides insight into the principles of Quantum Mechanics.

In this experiment where electromagnetic radiation strikes the surface of a metal, photons are emitted. This occurrence is what is known as the Photoelectric Effect. The energy of these photons can be determined by adding a electrical potential. As the voltage is incremented higher and higher, there will be a voltage where no more photons can reach the other plate. From this voltage, the maximum kinetic energy of the electrons can be found.

Classical theory leads to the belief that both the intensity of the light and the frequency of the light will increase the energy of the ejected electrons. However, observation shows that the kinetic energy of the photons emitted is independent of the intensity of the beam. Red light emitted no electrons regardless of intensity and increasing the intensity of the beam would increase the number of electrons emitted but not their energies.

Instead, energy increases linearly with the frequency of the light. Depending on the type of the metal, there will be a minimum frequency for which and electron will be emitted. The following equation summarizes this:

Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle hf = \phi + E_{k_{max}} \,}

where Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \phi} is the work function (a property of the metal), Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle h} is planck's constant, and Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle f} is the frequency.

All of this is significant because in classical theory there should be no threshold for electron emissions.

Compton effect

The Compton effect is a similar experiment to the photoelectric effect where they both involve photons interacting with something. But here, instead of a metal surface, the photon is incident on an electron, scattering it in a different direction.

Arthur H. Compton observed the scattering of x-rays from electrons in a carbon target and found scattered x-rays with a longer wavelength than those incident upon the target. The shift of the wavelength increased with scattering angle according to the Compton formula:

Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \lambda_f - \lambda_i = \frac{h}{m_e c}(1 - \cos \theta) \;}

Compton explained and modeled the data by assuming a particle (photon) nature for light and applying conservation of energy and conservation of momentum to the collision between the photon and the electron. The scattered photon has lower energy and therefore a longer wavelength according to the Planck relationship.

At a time (early 1920's) when the particle (photon) nature of light suggested by the photoelectric effect was still being debated, the Compton experiment gave clear and independent evidence of particle-like behavior. Compton was awarded the Nobel Prize in 1927 for the "discovery of the effect named after him".

Particle-Wave Duality and de Broglie Wavelength

Einstein proposed, in explaining the photoelectric effect, that light was not continuous, but that it was delivered in packets, known as photons. However this hypothesis failed to explain Young's Double Slit Experiment, in which light displayed wave-like properties (interference and diffraction). This led to the reasoning that light is not either particles or waves; it is somehow both particles and waves.

Louis de Broglie

This wave-particle duality of light led the French physicist Louis de Broglie to pose a question about particle-wave duality. Was this phenomenon only a property of light or is property of material objects as well? De Broglie suggested, lacking any experimental evidence in support of his hypothesis, that associated with any material particle moving with momentum Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle p} there is a wavelength Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \lambda} related to Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle p} according to

Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \lambda = \frac{h}{p}\!}

The wavelength Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \lambda} of a particle computed according to this equation is called its de Broglie wavelength.

Davission-Germer experiment

In 1927 de Broglie's hypothesis was experimentally proven with the Davisson-Germer experiment. Their experiment involved firing an electron beam at a nickel crystal and observing the scattering of the electrons. The scattering of these electrons would not be the same for all beam energies. As the energy of the beam increased the de Broglie wavelength of the electrons would shrink. At 54 eV the electrons would have a wavelength similar to that of X-rays and the scattering of the electrons were the same as those of the X-rays. The Davisson-Germer experiment demonstrated the wave nature of the electron.

Thompson-Reid experiment

As Davisson and Germer were going at it, another experiment took place: The Thompson-Reid experiment. Sir G.P. Thompson, working with his assistant A. Reid, took a thin gold foil, rolled it to be extremely flat, placed it in front of a 30 kV electron beam, and recorded the accompanying image on their photographic plate. The rings are associated with electron diffraction from the interplanar spacing within tiny crystallites in the gold foil. The continuous rings arise because the tiny crytallites are randomly oriented in the foil. Subsequently they took a sample of aluminium and did not roll it but worked carefully with it to obtain a crystalline sample. When this was exposed to their beam, spots lying around a ring-like structure were observed. This experiment demonstrated that electrons behave exactly as if they were waves.

It is interesting to recall that G.P. Thompson, who shared the 1937 Nobel Prize with Davisson for these experiments which proved that electrons are waves, is the son of J.J. Thompson who received the Nobel Prize in 1906 for proving that cathode rays were actually particles - electrons! And the amazing thing is that they were both right.

Uncertainty Principle

You cannot know both position and momentum simultaneously

Classical Wave Uncertainty

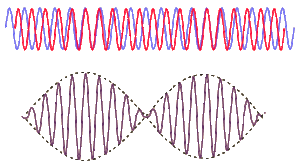
Superposition of two wave creates a beat pattern that helps to localize a wave

This uncertainty relationship stems from the difficulty in determining the position and wavelength of a classical wave simultaneously. Consider a sine wave. A sine wave repeats endlessly from Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle x = -\infty} to Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle x = \infty} . If you were to ask "Where is the wave located?" you would not be able to provide an answer since it is everywhere. However, if you add another wave of different wavelength you will begin to get a beat pattern, with periods of higher and lower amplitudes, as in the image to the right. This in essence allows you to localize the wave and answer the question about the location of the wave. Using this method we could continue adding waves until we have an exact position. However, since we are adding wave of different wavelength each time we are sacrificing the knowledge of wavelength. Mathematically we can represent this as

Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \Delta x \Delta k \sim 1\!}

where Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \Delta k} is the wave number of the wave and Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \Delta x} is the position of the wave. Using this same thought process we can formulate a similar uncertainty principle between the frequency, Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \Delta \omega} and time, Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \Delta t} properties of a wave.

Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \Delta \omega \Delta t \sin 1\!}

Heisenberg Uncertainty Principle

Werner Heisenberg

Werner Heisenberg applied the uncertainty principles in waves to de Broglie waves. Using the de Broglie relationship and the expression Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle k = \frac{2\pi}{\lambda}} we get an expression that relates the momentum of a particle to the wave number of its de Broglie wave.

Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle p = \frac{hk}{2\pi}\!} or using Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \hbar = \frac{h}{2\pi}} we have Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle p = \hbar k}

This implies that

Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \Delta k = \frac{\Delta p}{\hbar}\!}

relating this to the wave uncertainty we get

Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \Delta x \Delta p \sim \hbar\!}

This states that it is not possible to make a simultaneous determination of the position and the momentum of a particle with unlimited precision.

Similarly, we can extend this reasoning to the energy of photons (and any other canocally conjugated elements)

Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \Delta E\Delta t \sim \hbar\!}

which states that it is not possible to make a simultaneous determination of the energy and the time coordinate of a particle with unlimited precision.

Atomic Spectra

Bohr's model of an atom

Atomic Line Spectra

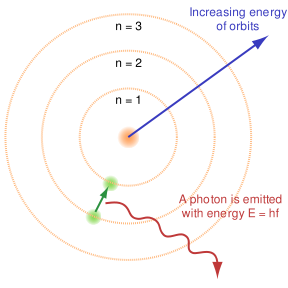
The atomic line spectra is the phenomena where photons are absorbed and emitted by atoms at characteristic frequencies. Classically, according to the Lorentz theory, an electron in orbit around a nucleus is accelerated, causing it to emit electromagnetic radiation. This must be accompanied by a decrease in kinetic energy of the electron, causing it to spiral into the nucleus and become unstable. This is not what happens however as the electrons can only orbit around the nucleus in specified orbits with defined energies. The energies at each level are given by:

Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle E=-hR/n^2}

Plum Pudding model of atom 1904

The plum pudding model of the atom by J.J. Thomson, who discovered the electron in 1897, was proposed in 1904 before the discovery of the atomic nucleus. In this model, the atom is composed of electrons (which Thomson still called "corpuscles," though G.J. Stoney had proposed that atoms of electricity be called electrons in 1894), surrounded by a soup of positive charge to balance the electron's negative charge, like negatively-charged "plums" surrounded by positively-charged "pudding". The electrons (as we know them today) were thought to be positioned throughout the atom, but with many structures possible for positioning multiple electrons, particularly rotating rings of electrons. Instead of a soup, the atom was also sometimes said to have had a cloud of positive charge.

Rutherford model of atom 1911 (AKA Planetary Model)

Rutherford's scattering experiment was called the famous Geiger-Marsden experiment in (1909), which suggested to Rutherford's analysis (1911) that the Plum pudding model was incorrect. Rutherford's new model for the atom, based on the experimental results, had a number of essential modern features, including a relatively high central charge concentrated into a very small volume in comparison to the rest of the atom and containing the bulk of the atomic mass (the nucleus of the atom), and a number of tiny electrons circling around the nucleus like planets around the sun.

Planetary model of Atom contradicts experiments

Stability of matter: why doesn't everything fall to the center of the nucleus?
Atomic Spectra lines: why can't the electron's orbit be smaller?

Bohr's Model of atom 1913

Basic hypothesis
Electrons in atoms follow classical physics and Rutherford's atomic model
An electron jumping the specified orbital levels will either emit or absorb electromagnetic radiation
This was great for explaining the Rydberg formula for the emission of spectral lines

Bohr's theory applied to hydrogen-like atoms

It was a much simplified version of the actual atom
It could not be applied to larger atoms

Line Spectra (Rydberg's formula)

Rydberg's formula for Hydrogen:

Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \frac{1}{\lambda_{\mathrm{vac}}} = R_{\mathrm{H}} \left(\frac{1}{n_1^2}-\frac{1}{n_2^2}\right)}

where

Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \lambda_{\mathrm{vac}}} is the wavelength of the light emitted in vacuum,

Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle R_{\mathrm{H}}} is the Rydberg constant for hydrogen,

Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle n_1} and Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle n_2} are integers such that Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle n_1 < n_2} ,

By setting Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle n_1} to 1 and letting Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle n_2} run from 2 to infinity, the spectral lines known as the Lyman series converging to 91 nm are obtained, in the same manner:

$n_{1}$	$n_{2}$	Name	Converge toward
1	$2\rightarrow \infty$	Lyman series	91.13 nm
2	$3\rightarrow \infty$	Balmer series	364.51 nm
3	$4\rightarrow \infty$	Paschen series	820.14 nm
4	$5\rightarrow \infty$	Brackett series	1458.03 nm
5	$6\rightarrow \infty$	Pfund series	2278.17 nm
6	$7\rightarrow \infty$	Humphreys series	3280.56 nm

The Lyman series is in the ultraviolet while the Balmer series is in the visible and the Paschen, Brackett, Pfund, and Humphreys series are in the infrared.

Chemical bonds between atoms were now explained, by Gilbert Newton Lewis in 1916, as the interactions between their constituent electrons. As the chemical properties of the elements were known to largely repeat themselves according to the periodic law, in 1919 the American chemist Irving Langmuir suggested that this could be explained if the electrons in an atom were connected or clustered in some manner. Groups of electrons were thought to occupy a set of electron shells about the nucleus.

The Stern–Gerlach experiment of 1922 provided further evidence of the quantum nature of the atom. When a beam of silver atoms was passed through a specially-shaped magnetic field, the beam was split based on the direction of an atom's angular momentum, or spin. As this direction is random, the beam could be expected to spread into a line. Instead, the beam was split into two parts, depending on whether the atomic spin was oriented up or down.

The Schrödinger model of the atom

In 1926, Erwin Schrödinger, using Louis de Broglie's 1924 proposal that particles behave to an extent like waves, developed a mathematical model of the atom that described the electrons as three-dimensional waveforms, rather than point particles. A consequence of using waveforms to describe electrons is that it is mathematically impossible to obtain precise values for both the position and momentum of a particle at the same time; this became known as the uncertainty principle, formulated by Werner Heisenberg in 1926. In this concept, for each measurement of a position one could only obtain a range of probable values for momentum, and vice versa. Although this model was difficult to visualize, it was able to explain observations of atomic behavior that previous models could not, such as certain structural and spectral patterns of atoms larger than hydrogen. Thus, the planetary model of the atom was discarded in favor of one that described atomic orbital zones around the nucleus where a given electron is most likely to exist.

Atomic Shell Structure

Quantum numbers: The quantum state of an electron is determined by its quantum number

Quantum Number	Symbol	Orbital meaning	Range of values	Value example
Principal	$n\$	shell	$1\leq n\,\!$	$n=1,2,3...\,\!$
Orbital (Angular momentum)	$\ell \$	subshell	$(0\leq \ell \leq n-1)\$	for $n=3\,\!$ : $\ell =0,1,2\,(s,p,d)\$
Magnetic (Projection of angular momentum)	$m_{\ell }\$	energy shift	$-\ell \leq m_{\ell }\leq \ell \$	for $\ell =2\$ : $m_{\ell }=-2,-1,0,1,2\,\!$
Spin projection	$m_{s}\,\!$	spin	$-{\begin{matrix}{\frac {1}{2}}\end{matrix}},{\begin{matrix}{\frac {1}{2}}\end{matrix}}\$	for an electron, either: $-{\begin{matrix}{\frac {1}{2}}\end{matrix}},{\begin{matrix}{\frac {1}{2}}\end{matrix}}\$

Selection rule in quantum transitions $N=\pm 1$ ^[2]

Electron configurations ^[3]

Pauli exclusion principle ^[4]

Periodic table ^[5]

Schrödinger Equation

Erwin Schrodinger

For 1 particle in 3D, the time-dependent Schrödinger Equation is as follows

i\hbar {\frac {\partial }{\partial t}}\Psi (\mathbf {r} ,\,t)=-{\frac {\hbar ^{2}}{2m}}\nabla ^{2}\Psi (\mathbf {r} ,\,t)+V(\mathbf {r} )\Psi (\mathbf {r} ,\,t)

where

$\mathbf {r} =(x,y,z)$ is the particle's position in three-dimensional space,
$\Psi (\mathbf {r} ,t)$ is the wavefunction, which is the amplitude for the particle to have a given position r at any given time t.
$m$ is the mass of the particle.
$V(\mathbf {r} )$ is the potential energy of the particle at each position r.

This describes many things but not waves at a very high energy, and as such further relativistic derivations were reconciled by Dirac and others later in the 20th century.

References

↑ http://www.youtube.com/watch?v=8GZdZUouzBY 1927 Solvay Physics Conference
↑ http://hyperphysics.phy-astr.gsu.edu/hbase/quantum/hydazi.html#c3 Selection Rules for Electronic Transitions
↑ http://en.wikipedia.org/wiki/Atomic_electron_configuration_table Atomic electron configuration table at Wikipedia
↑ http://hyperphysics.phy-astr.gsu.edu/HBASE/Pauli.html Pauli Exclusion Principle at Hyperphysics
↑ http://en.wikipedia.org/wiki/Periodic_table_(electron_configurations) The Periodic table of electron configurations at Wikipedia

Description of Modern Physics - Real Wikipedia & Syllabus written by Ingo Wiedenhover
Krane, Kenneth Modern Physics

[1] ttp://www.youtube.com/watch?v=8GZdZUouzBY 1927 Solvay Physics Conference

[2] ttp://hyperphysics.phy-astr.gsu.edu/hbase/quantum/hydazi.html#c3 Selection Rules for Electronic Transitions

[3] ttp://en.wikipedia.org/wiki/Atomic_electron_configuration_table Atomic electron configuration table at Wikipedia

[4] ttp://hyperphysics.phy-astr.gsu.edu/HBASE/Pauli.html Pauli Exclusion Principle at Hyperphysics

[5] ttp://en.wikipedia.org/wiki/Periodic_table_(electron_configurations) The Periodic table of electron configurations at Wikipedia

[1]

[2]

[3]

[4]

[5]

PHZ3400-09 Modern Physics

Contents

Introduction