Solution to Set 4

Problem 1

Cu, density $\rho =8.885{g \over cm^{3}}$, atomic mass $m_{a}=63.57amu$, fcc structure

a. In $1m^{3}$, the number of moles is $({8.885g \over 1cm^{3}})({1cm^{3} \over 5\times 10^{6}m^{3}})({1mol \over 63.55g})=1.40\times 10^{5}{mol \over m^{3}}$

b. Atoms in $1m^{3}$ $({1.40\times 10^{5}mol \over 1m^{3}})({6.022\times 10^{23} \over 1mol})=8.43\times 10^{28}atoms$

c. Since the bonds between the atoms are small compared to the diameter of the atom, we neglect the bonds for an estimate. The structure is fcc and the length of each side of the cube is $2r=362pm$, where r is the radius of one Cu atom.

d. Atomic radius

$p={m_{a} \over V_{s}}$, where $V_{s}={4 \over 3}\pi r^{3}$

$p={m_{a} \over {4 \over 3}\pi r^{3}}$

$r=({3m_{a} \over 4p\pi })^{1 \over 3}=128pm$

e. Mass of 1 atom

$(63.57amu)({1.66\times 10^{-24}g \over 1amu})=1.06\times 10^{-22}g$

Problem 2

Draw the planes whose Miller indices are (421), (112), (1-10) and (-121). State their intercepts on the x- y- and z- axes.

Miller indicies are used as a way to note planes and directions in crystal lattices. To graph the miller indicies in a cartesian coordinate system, you can use the equation $1/MillerIndex=Intercept$

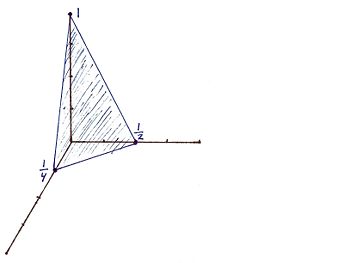
For the Miller indicies (421) the x, y, and z intercepts are respectively (1/4, 1/2, 1). This gives rise to the graph

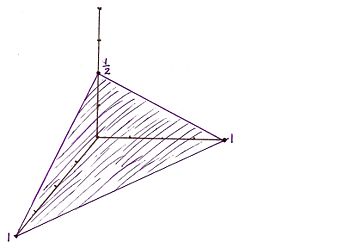
For the Miller indicies (112) the x, y, and z intercepts are respectively (1, 1, 1/2). This gives rise to the graph

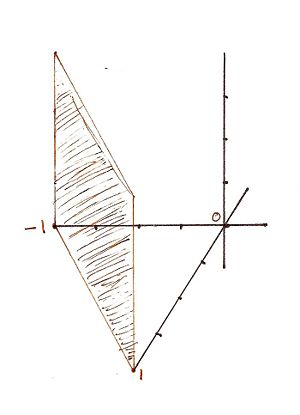
For the Miller indicies (1-10) the x, y, and z intercepts are respectively (1, -1, 0) meaning that there is no intercept on the z axis. This gives rise to the graph

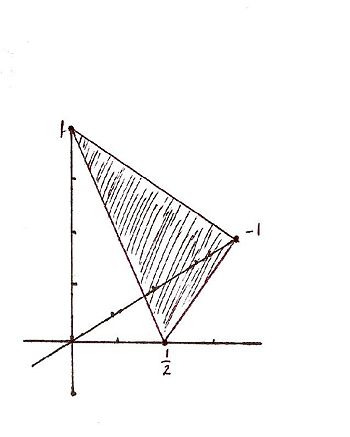
For the Miller indicies (-121) the x, y, and z intercepts are respectively (-1, 1/2, 1). This gives rise to the graph

Problem 3

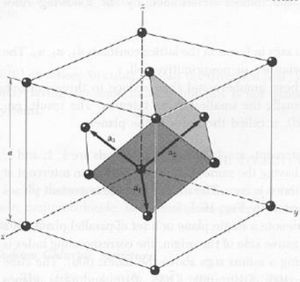
a. fcc and primitive cell

b. $V_{fcc}=lwh=a_{1}a_{2}a_{3}={1 \over 2}a(x+y){1 \over 2}a(y+z){1 \over 2}a(z+x)={1 \over 8}a^{3}({1 \over 2}+{1 \over 2})(1+1)({1 \over 2}+{1 \over 2})={1 \over 4}a^{3}$

The volume of the primitive cell is ${1 \over 4}$ the volume of the fcc cell.

c. The FCC cell has 4 atoms in it. Each vertex is 1/4 of an atom, and each face cell is 1/2 an atom in the cell. The Primitive Cell has 1 atom in it as it is the smallest possible cell to get. Each vertex of it has 1/8 of an atom on it.