Self-organized criticality and earthquakes: Difference between revisions
No edit summary |
No edit summary |
||
| Line 2: | Line 2: | ||
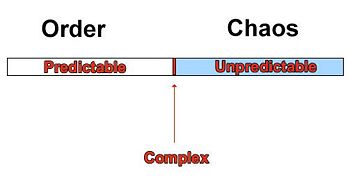
In 1987 the team of Bak, Tang, and Weisenfeld presented a paper on "Self-organized Criticality" which hoped to provide a very simplified model to explain the complexity that is found in nature. Prior to this, many models describing complex systems had been developed however there was no general theory of complexity built on a strong mathematical foundation. The theory with which the team attempted in their paper explains the "self-organized critical state" (SOC) by comparing it to that of a steep sand pile which emits avalanches of all sizes as more sand is added to the system. This state is characterized by the fact that the system has self-organized itself to a point where it is on the border between predictability and unpredictability to the so called "edge of chaos." For the specific case of earthquakes, it can be postulated that the crust of the earth is a highly self-organized system which features earthquakes that are unpredictably strong, where the intensity and frequency of the quakes follow a power law distribution. Power law distributions are a key aspect of SOC. | In 1987 the team of Bak, Tang, and Weisenfeld presented a paper on "Self-organized Criticality" which hoped to provide a very simplified model to explain the complexity that is found in nature. Prior to this, many models describing complex systems had been developed however there was no general theory of complexity built on a strong mathematical foundation. The theory with which the team attempted in their paper explains the "self-organized critical state" (SOC) by comparing it to that of a steep sand pile which emits avalanches of all sizes as more sand is added to the system. This state is characterized by the fact that the system has self-organized itself to a point where it is on the border between predictability and unpredictability to the so called "edge of chaos." For the specific case of earthquakes, it can be postulated that the crust of the earth is a highly self-organized system which features earthquakes that are unpredictably strong, where the intensity and frequency of the quakes follow a power law distribution. Power law distributions are a key aspect of SOC. | ||
Some of the criticisms of the theory include that it does not mathematically show why the critical systems follow a power law distribution and it also does not explain why the systems self organize. | |||
==The Sandpile Paradigm== | ==The Sandpile Paradigm== | ||
Revision as of 16:38, 21 April 2009
In 1987 the team of Bak, Tang, and Weisenfeld presented a paper on "Self-organized Criticality" which hoped to provide a very simplified model to explain the complexity that is found in nature. Prior to this, many models describing complex systems had been developed however there was no general theory of complexity built on a strong mathematical foundation. The theory with which the team attempted in their paper explains the "self-organized critical state" (SOC) by comparing it to that of a steep sand pile which emits avalanches of all sizes as more sand is added to the system. This state is characterized by the fact that the system has self-organized itself to a point where it is on the border between predictability and unpredictability to the so called "edge of chaos." For the specific case of earthquakes, it can be postulated that the crust of the earth is a highly self-organized system which features earthquakes that are unpredictably strong, where the intensity and frequency of the quakes follow a power law distribution. Power law distributions are a key aspect of SOC.
Some of the criticisms of the theory include that it does not mathematically show why the critical systems follow a power law distribution and it also does not explain why the systems self organize.
The Sandpile Paradigm
Consider a pile of sand of any size. As sand is added to the pile(at any position not just limited to the center) the size and slope of the pile will become larger and larger. Soon it will reach a slope where the addition of more sand will trigger avalanches that will increase in size as the slope of the pile also increases in size. Eventually this slope will reach a particular size where it will no longer increase due to the fact the amount of sand added will balance out with the amount of sand leaving the pile. This is known as the critical stationary state of the sandpile. At this point the individual dynamics of each grain of sand is not as important as a new emergent dynamic of the sandpile has become global. Any perturbation made to the system may cause avalanches that are either large or small perhaps causing the physical appearance of the sandpile to change but this system dynamic will remain complex. The same perturbation that might cause a small avalanche could also cause a large avalanche.
The Sandpile Model

The best way to understand this is to consider a very simple model which can be easily recreated and simulated using a computer. Imagine a sandpile with equal sized sand granules that have no imperfections (to simplify the interaction between each sand granule and the ones next to it. As Bak puts it this is "theoretical physicist's sand". Sand is then randomly added to a site in the growing pile and thus increasing its height. When a particular site becomes too high it will distribute the sand at that site to the 4 adjacent sites around it. So if the highest a particular site can reach is 4 units of sand then those 4 units will be taken away from that site and 1 will be given to each of the 4 adjacent sites.
To better understand what is occurring refer to the very useful applet that was created by Maslov.
Figure 3 on the left also shows the result of the domino effect that takes place when an avalanche begins.
The 'edge of chaos' and power law distributions
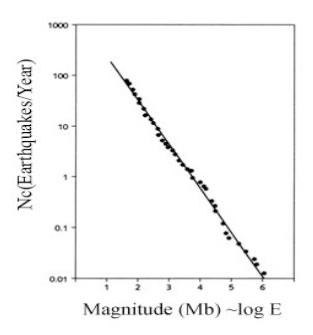
The Gutenberg-Richter Law
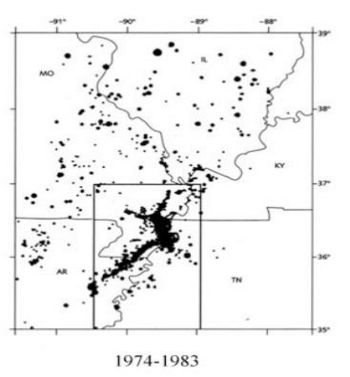
The crust of the Earth is in a self-organized critical state
Sources
Self-Organized Criticality: Emergent Complex Behavior in Physical and Biological Systems. Cambridge Lecture Notes in Physics Part 10.
How Nature Works: The Science of Self-Organized Criticality. Per Bak.
Modeling Extinction. M.E.J. Newman, R.G. Palmer. Santa Fe Institute Studies in the Sciences of Complexity.
Introduction to Self-Organized Criticality & Earthquakes by Nathan Winslow. http://www.econ.iastate.edu/classes/econ308/tesfatsion/SandpileCA.Winslow97.htm