Solution to Set 4

Problem 1

Cu, density $\rho =8.885{g \over cm^{3}}$, atomic mass $m_{a}=63.57amu$, fcc structure

a. In $1m^{3}$, the number of moles is $({8.885g \over 1cm^{3}})({1cm^{3} \over 5\times 10^{6}m^{3}})({1mol \over 63.55g})=1.40\times 10^{5}{mol \over m^{3}}$

b. Atoms in $1m^{3}$ $({1.40\times 10^{5}mol \over 1m^{3}})({6.022\times 10^{23} \over 1mol})=8.43\times 10^{28}atoms$

c. Since the bonds between the atoms are small compared to the diameter of the atom, we neglect the bonds for an estimate. The structure is fcc and the length of each side of the cube is $2r=362pm$, where r is the radius of one Cu atom.

d. Atomic radius

$p={m_{a} \over V_{s}}$, where $V_{s}={4 \over 3}\pi r^{3}$

$p={m_{a} \over {4 \over 3}\pi r^{3}}$

$r=({3m_{a} \over 4p\pi })^{1 \over 3}=128pm$

e. Mass of 1 atom

$(63.57amu)({1.66\times 10^{-24}g \over 1amu})=1.06\times 10^{-22}g$

Problem 2

Draw the planes whose Miller indices are (421), (112), (1-10) and (-121). State their intercepts on the x- y- and z- axes.

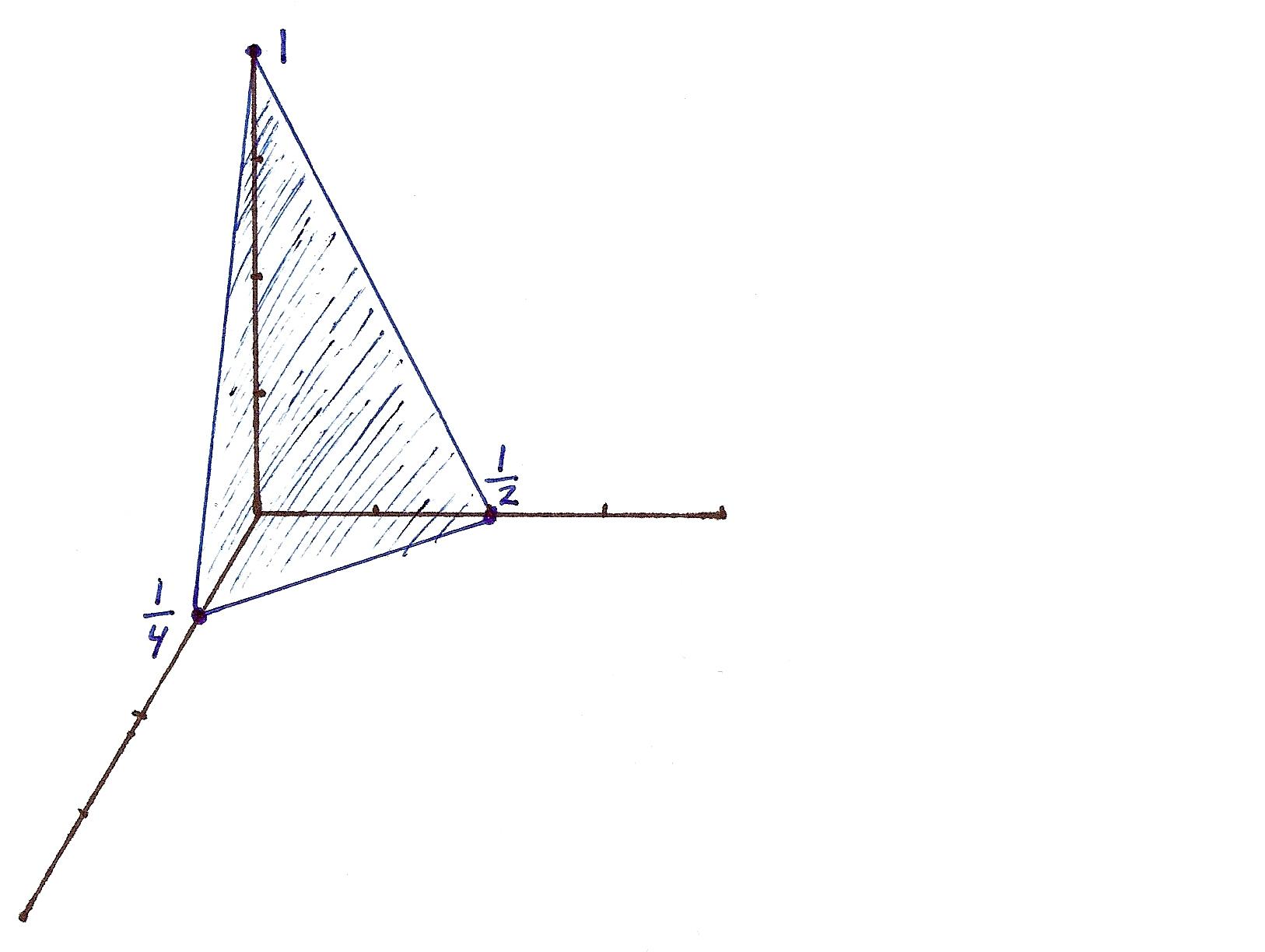
For the Miller indicies (421) the x, y, and z intercepts are respectively (1/4, 1/2, 1). This gives rise to the graph

For the Miller indicies (1-10) the x, y, and z intercepts are respectively __________.

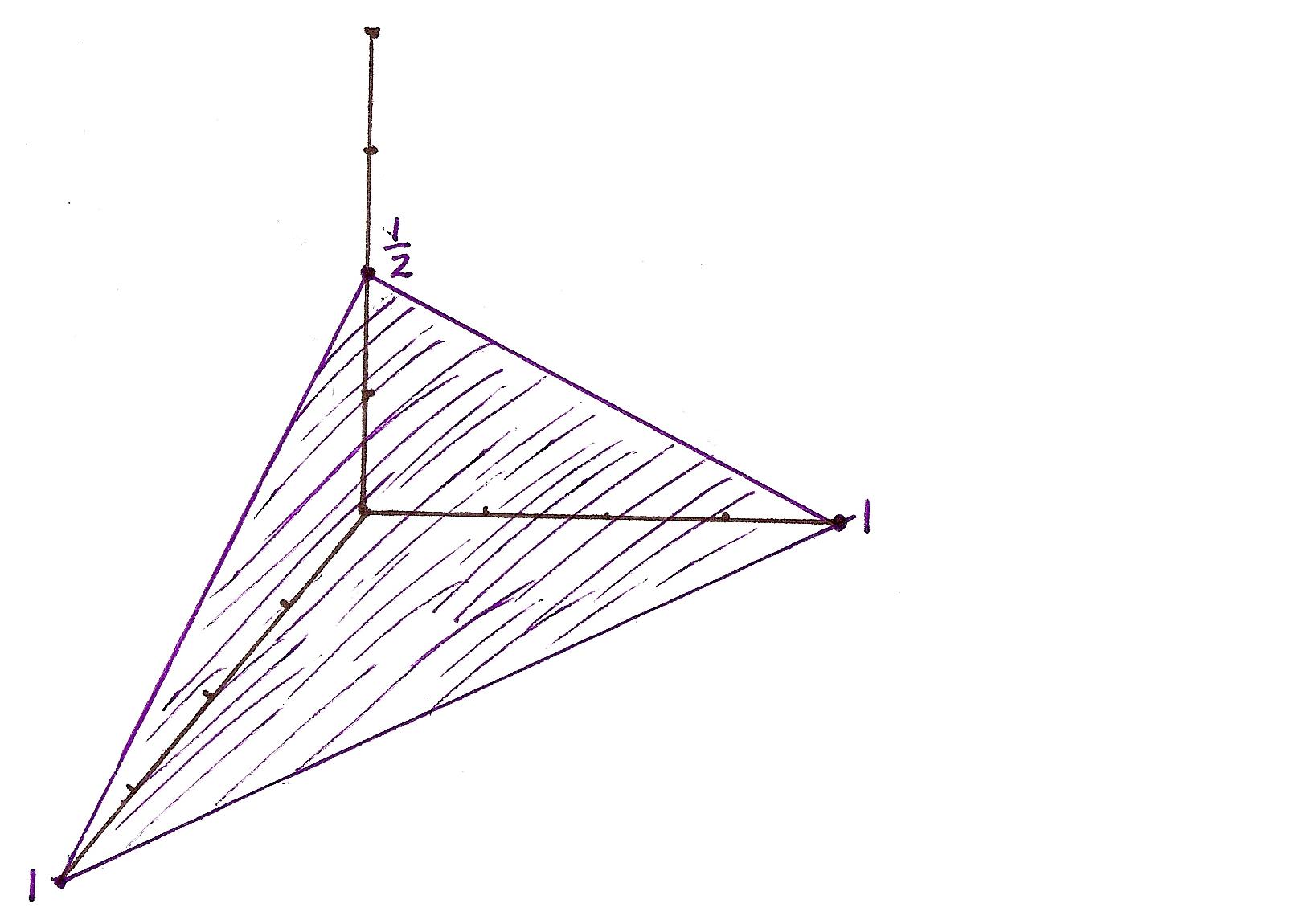
For the Miller indicies (112) the x, y, and z intercepts are respectively (1, 1, 1/2). This gives rise to the graph

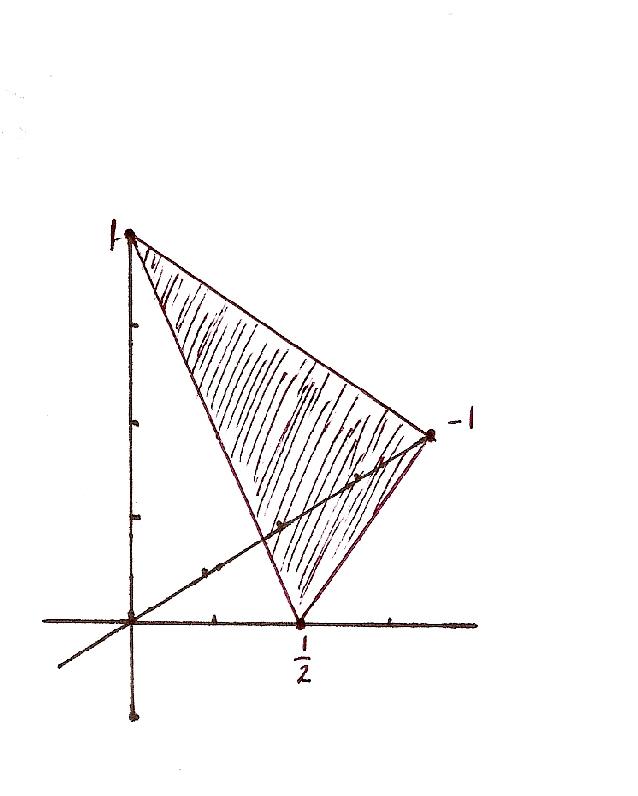
For the Miller indicies (-121) the x, y, and z intercepts are respectively (-1, 1/2, 1). This gives rise to the graph

Problem 3

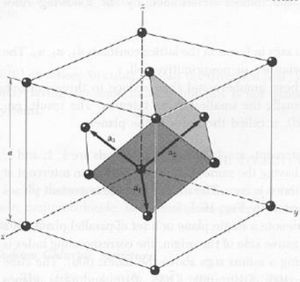
a. fcc and primitive cell

b. $V_{fcc}=lwh=a_{1}a_{2}a_{3}={1 \over 2}a(x+y){1 \over 2}a(y+z){1 \over 2}a(z+x)={1 \over 8}a^{3}({1 \over 2}+{1 \over 2})(1+1)({1 \over 2}+{1 \over 2})={1 \over 4}a^{3}$

The volume of the primitive cell is ${1 \over 4}$ the volume of the fcc cell.

c. There are 4 atoms in the fcc cell and 6 in the primitive cell. There are 6 because there is 1 atom per vertex in the primitive cell while the fcc cell has 2 atoms per vertex and one additional atom per face.