Homeworks 1

Homeworks 1

Homeworks 1 About this Assignment Topics Sizes Algebra Conversion Solar Systems Elliptical orbits Chapters 1 - 2 Lectures 2 - 4

Homeworks 1 is the attempted solution to our second task in the course Introduction to Astrophysics. This assignment is due on Wednesday 09/09/09 and was assigned on 09/01/09. Solutions were created by Group 1 (RyanT, KimW, SaraC, ZackM, TiaraD) to be completed by 09/11/09.

This assignment covers Chapter 1 and 2 in the book, Lectures 2 through 4. The topics in this assignment range from sizes, to solar systems, to why the orbits of the planets are elliptical.

Problem 1

List in order of increasing size and give the approximate size of the following objects: An atom, a biological cell, a cluster of galaxies, the Earth, a galaxy, the Local Group of galaxies, a neutron, a neutron star, a person, the Solar System, our sun. Note: you may have to look in other books besides your textbook to get all this information.

There are 3 ways to approach this problem: By radius, by volume, and by mass.

This problem coincides with Lecture #2. 

By Radius

1. Neutron = Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle 3 quarks \;} (or Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle r \approx 1 fm \;} = Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle 1.11 \times 10^{-15} m \;} )
2. Atom = empirical atomic radius Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle r \approx 62 - 520 pm \;} = Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle 0.62 - 5.20 \times 10^{-10} m \;}
3. Biological Cell = Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle 10 \mu m \;} = Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle 1.00 \times 10^{-5}}
4. Person = height = 4'11" = Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle 1.50 m \;}
5. Neutron Star = Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle 12 km \;} = Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle 1.20 \times 10^4 m \;}
6. Earth = radius of the Earth = Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle 6378.1 km \;} = Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle 6.38 \times 10^6 m \;}
7. Sun = radius of the Sun = Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle 695500 km \;} = Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle 6.96 \times 10^8 m \;}
8. Solar System = radius of the comet Oort Cloud's orbit = Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle 7.5 \times 10^{12} km \;} = Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle 7.50 \times 10^{15} m \;}
9. Galaxy = radius of most galaxies = Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle 50000 ly \;} = Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle 4.73 \times 10^{20} m \;}
10. Local Group of Galaxies = radius of Local Group = Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle 5 \times 10^6 ly \;} = Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle 4.73 \times 10^{22} m \;}
11. Cluster of Galaxies = approximate size of most clusters = Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle 5 Mpc \;} = Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle 1.54 \times 10^{23} m}

By Volume

To be Completed

By Mass

To be Completed

Problem 2

The nearest star outside the solar system is about 4 light years away.

1. How far away is the star in kilometers?
2. Suppose you travel to the nearest star in a rocket ship moving at 100 km per hour (100 km/hr is

about 62 mi/hr, a typical automobile speed on a Florida highway). How many years will it take you to get to the star?

1. Suppose you travel to the star at 10 km per second (the speed of a rocket in orbit around the Earth). How many years will it take you to get to the star?

1. One light year is approximately 9.460730472 x 10^15 meters. Therefore, this star is approximately

   3.784 x 10^13 kilometers.

2. Knowing the distance of this star in kilometers, one can find the time it takes to get there with t = d/v.

   t = (3.784 x 10^13km)/(100km/hr) = 3.784 x 10^11 hours.  This equates to about 43,196,347 years!

3. Again, use t = d/v, but with time in seconds. t = (3.784 x 10^13km)/(10km/s) = 3.784 x 10^12 seconds.

   This equates to about 120,000 years!

Problem 3

Use the size of the Astronomical Unit in kilometers and the length of the year in seconds to calculate how fast the Earth moves in its orbit in kilometers/second.

Problem 4

Describe the essential differences between the Ptolemaic, Copernican, and Keplerian descriptions of planetary motion.

The Ptolemaic description of planetary motion was that of a geocentric solar system in which all planets and the sun orbit the earth. Ptolemy also described the retrograde motion of the planets and moon using epicycles.

Copernicus introduced the heliocentric model of the solar system in which all plnets orbit the sun. Copernicus also explained that the apparent retrograde motion of the planets and moon is actually a result of the earth's motion.

Kepler maintained the heliocentric model of planetary motion and introduced that planets move in elliptical orbits about the sun with his idea of conic sections.

Problem 5

Use Newton’s laws to show that the orbits of planets are ellipses.

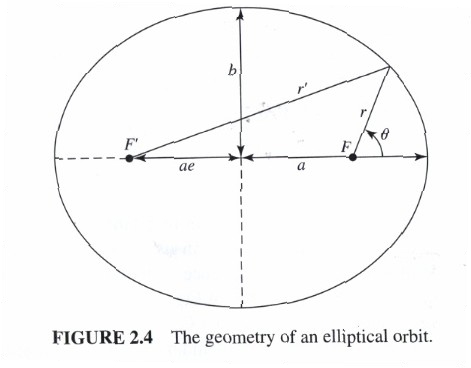
Kepler's First Law is that the orbits of our planets are ellipses (with the sun at one focal point). However it does not explain why the planets move as they do in elliptical loops. Therefore, this question can be restated: Use Newton's laws to derive Kepler's First Law.

This problem coincides with Chapter 2.3 Kepler's Laws Derived and Lecture #4. 

where

Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle a \;} = semimajor axis

Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle b \;} = semiminor axis

Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \textbf{r} \;} and Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \textbf{{r}'} \;} = distances to focal points

Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \textbf{F} \;} and Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \textbf{{F}'} \;} = focal points

Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle e = \frac{\left | F - {F}' \right |}{2a} \;} = eccentricity

This problem is incomplete.

The Equation for an Ellipse is Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle r + {r}' = 2a \;} , and in polar coordinates

Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle r = \frac{a(1-e^2)}{1+e\cos\theta} \;} for Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle 0 \leq e < 1}

Conservation of Angular Momentum Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \textbf{L} \;}

Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \textbf{L} = m_1 \textbf{r}_1 \times \textbf{v}_1 + m_2 \textbf{r}_2 \times \textbf{v}_2 \;}

Define Reduced Mass Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \mu \;}

Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \mu \equiv \frac{ m_1 m_2 }{m_1 + m_2} \;}

Total Angular Momentum Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \textbf{L} \;} with respect to Reduced Mass Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \mu \;}

Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \textbf{L} = \mu \cdot \textbf{r} \times \textbf{v} \;}

With reduced mass Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \mu \;} (and because of it), total angular momentum Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \textbf{L} \;} is constant.

Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \frac{d \textbf{L}}{dt} = 0 \;}

Kepler's 1st Law

Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle r = \frac{\tfrac{L^2}{\mu^2}}{GM(1+e\cos\theta)} \;}

References

• B.W. Carroll & D. A. Ostlie (2007). An Introduction to Modern Astrophysics (Chapter 2). Addison Wesley. ISBN 0-8053-0402-9
• Lecture #2
• Lecture #3
• Lecture #4