Homeworks 3: Difference between revisions
No edit summary |
|||
| Line 98: | Line 98: | ||
==Problem 5== | ==Problem 5== | ||
Estimate the size of the spherical | Estimate the size of the spherical aberration of a spherical mirror of 1m-diameter and a focal | ||
length of 2 meter. (Hint: Calculate the size of the smeared image of a star at the focal point and | length of 2 meter. (Hint: Calculate the size of the smeared image of a star at the focal point and | ||
compare it to the size (in arc-sec) of an extended object, and remember your PHY2048/9). | compare it to the size (in arc-sec) of an extended object, and remember your PHY2048/9). | ||
Though I will be unable to draw diagrams for this problem, the equations that come from them are fairly obvious. | |||
We all know that for small angles, <math> f=\frac{R}{2} </math> where f is the focal length and R is the radius of curvature for a spherical mirror. The problem is that with large angles, the foci will not match up, causing the aberration. | |||
For large angles, and by drawing a diagram the focal length can be written as, | |||
<math> f=x+y </math>, and the angles can be determined directly through trigonometric relations. They are, <math> \cos{\phi}=\frac{R-y}{R} </math> and <math> \tan{\theta}=\frac{h}{x} </math>. Here I define, <math> \theta=2\phi </math>. | |||
more to come. | |||
Revision as of 12:23, 25 September 2009
Homework 3 due on 09/21/2009
Problem 1
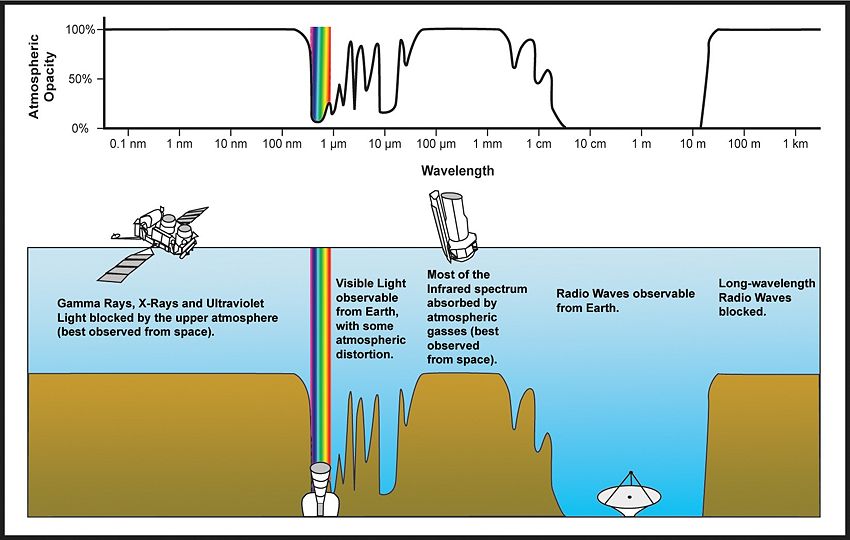
For each of the following types of electromagnetic radiation give a typical wavelength and state whether it can penetrate the Earth’s atmosphere: radio, microwave, infrared, visible, ultraviolet, X-ray, Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \gamma} -ray.
| Wavelength | Wavelength Range | Does it penetrate Earth's Atmosphere? |
|---|---|---|
| Radio | 10 m - Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle 10^8} m | From ~10 m to ~20 m, yes |
| Microwave | Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle 10^{-3}} m - 10 m | Some is absorbed at smaller wavelengths |
| Infrared | 700 nm - Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle 10^{-3}} m | Most are absorbed by atmospheric gasses |
| Visable | 400 nm - 700 nm | Yes, with some distortion |
| Ultraviolet | Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle 10^{-8}} m - 400 nm | No, all incoming rays are blocked |
| X-Ray | Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle 10^{-11}} m - Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle 10^{-8}} m | No, all incoming rays are blocked |
| Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \gamma} -ray | Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle 10^{-17}} m - Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle 10^{-11}} m | No, all incoming rays are blocked |
Problem 2
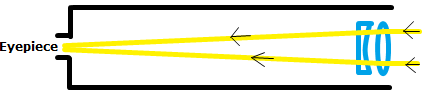
Sketch the typical light path of reflector and refractor telescopes, and name two of the advantages and disadvantages for each of the designs. Catadioptric designs such as the Maksukov and Schmidt telescopes have both a primary lense and a mirror. Name the main advantage and drawback of these designs, and their main application.
Refraction Telescope
| Advantages | Disadvantages |
|---|---|
| High resolution | Large objective lenses deform under gravity. |
| No incoming light is blocked. | Chromatic aberration |
Reflection Telescope
| Advantages | Disadvantages |
|---|---|
| No chromatic aberration | The prime focus is in the path of incoming light. |
| Only the reflecting surface needs to be polished to perfection. | Large detectors produce a lot of torque. |
Catadioptric Telescope
Catadioptric telescopes like the Schmidt telescope provide a wide-angle field of view and thus are often used as cameras. However, they suffer from both spherical and chromatic aberration.
Problem 3
Name and explain in your own words three of the optical artifacts of spherical mirrors. Explain why telescopes with multiple-mirrors such as Keck or the upcoming space based NGST are inferior for direct imaging of planets compared to mono-mirror systems. Why, then, are all large telescope systems are based on multiple mirror designs?
Spherical mirrors suffer from several types of optical artifacts. Coma is an effect that causes point sources to appear elongated. Astigmatism occurs when an image is focused onto slightly different points on the focal plane. Curvature of field occurs due to the fact that images are focused on a curve rather than on a plane.
Telescopes with multiple mirrors are inferior for direct imaging of planets because of the signal noise induced by the edges of the mirrors. However, most large telescopes continue to use multi-mirror designs since it is much easier to support several small mirrors rather than one large mirror.
Problem 4
Use the Rayleigh Criterion to estimate the diffraction limit (in arc sec) for the angular resolution of a typical 1m class telescope in the near IR (2 μm), and discuss the result.
The Rayleigh Criteron: Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \theta \thickapprox 1.22 \frac{\lambda}{D} }
Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \lambda = 2\times10^{-6}m }
Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle D=1m}
We plug in these values and we get that:
Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \theta \thickapprox 1.22 \frac{2\times10^{-6}m}{1m} = 2.44\times10^{-6}}
Problem 5
Estimate the size of the spherical aberration of a spherical mirror of 1m-diameter and a focal length of 2 meter. (Hint: Calculate the size of the smeared image of a star at the focal point and compare it to the size (in arc-sec) of an extended object, and remember your PHY2048/9).
Though I will be unable to draw diagrams for this problem, the equations that come from them are fairly obvious.
We all know that for small angles, Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle f=\frac{R}{2} } where f is the focal length and R is the radius of curvature for a spherical mirror. The problem is that with large angles, the foci will not match up, causing the aberration.
For large angles, and by drawing a diagram the focal length can be written as, Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle f=x+y } , and the angles can be determined directly through trigonometric relations. They are, Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \cos{\phi}=\frac{R-y}{R} } and Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \tan{\theta}=\frac{h}{x} } . Here I define, Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \theta=2\phi } .
more to come.