PHZ3400-09 Modern Physics

“ Ah, the reason we all majored in physics in the first place... ”

Group 3 is the best

Modern Physics Society of Physics Students Atom Header Bold Text Italic text Bold Italics Normal Text

Modern Physics (Greek: physis – φύσις meaning "nature") refers to the branches of physics developed from the discovery of the quantum nature of physics at the beginning of the 20th century. More generally, the term can refer to any branch of physics developed from this time period onward.

Modern Physics introduces the exciting developments of physics in the 20th Century (and some of the 21st). Well, anything that happened after PHY2049. We will see how the seeds of this great revolution were planted in the greatest triumph of the 19th century physics! And a revolution it was! There is no other way to describe the fascinating, surprising, and mind boggling discoveries of relativity, quantum mechanics, and the structure of matter. Some of the great scientists we will encounter along the way are Planck, Einstein, Curie, Rutherford, Bohr, Pauli, Dirac, Schrodinger, Heisenburg, Fermi, Geynman, Rydberg, and Schrieffer. Two of these giants of the 20th century physics, Dirac and Schrieffer, have served on the FSU faculty!

Introduction

• Notes: Lecture
• Lecturer: Dr. Vlad's Friend
• Time: 12:20pm - 1:10pm
• Dates: Wednesday, January 14, 2009 & Friday, January 16, 2009
• Place: The Classroom Building, HCB 317, Florida State University

“ At the end of the 19th century, scientists had gotten quite a lot done. But there was no explanation for things that happened at the quantum level. What happened when things got very small and very fast? ”

Black Body Radiation

Intensity vs. Wavelength

• Blackbody absorbs / not emits light
• Stefan Boltzmann Law

$I=\sigma T^{4}$

where

I = black-body irradiance, energy flux density, radiant flux, or the emissive power

T = the black body's thermodynamic temperature (also called absolute temperature)

σ = Stefan–Boltzmann constant = :$\sigma ={\frac {2\pi ^{5}k^{4}}{15c^{2}h^{3}}}=5.670400\times 10^{-8}{\textrm {J\,s}}^{-1}{\textrm {m}}^{-2}{\textrm {K}}^{-4}$

• Wien's Law

$\lambda _{\mathrm {max} }*{T}={b}$

where

λ_max = peak wavelength in meters

T = Temperature of the blackbody in Kelvins

b = Wien's displacement proportionality constant = Template:Val

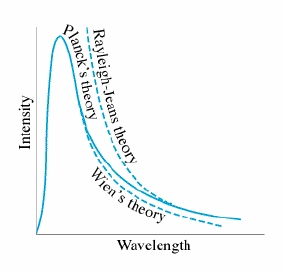
• Blackbody Radition Spectrum: UV catastrophe - Classical physics does not explain this
• This led to Planck's Hypothesis

Planck's Hypothesis

• radiation comes from electric oscillators
• energy of each mode of oscillation is quantized: $E=nhv$
• Energy can be emitted or absorbed

Specific Heat of Solids

• Cv / Na = const
• Cv = 3 Na K from classical physics
• But at low temperatures C drops sharply and approaches zero
• Phonons: quantization of lattice vibrations

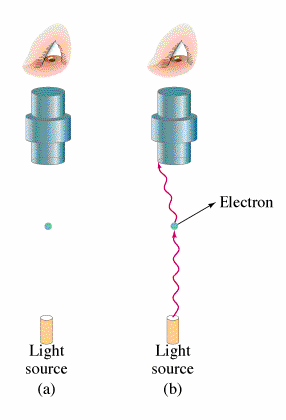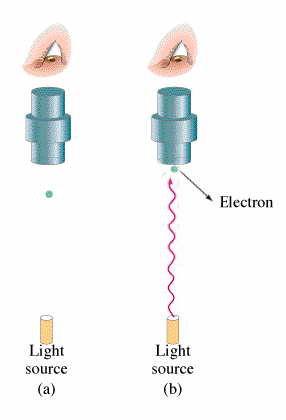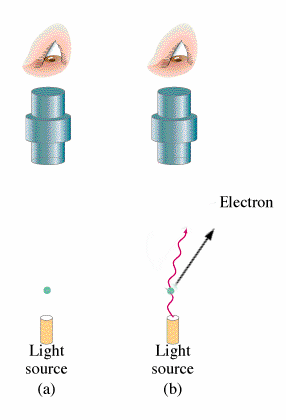
Photoelectric Effect

If Nobel prizes were given on the basis of popularity of the work alone then it would make no sense as to why Einstein won the Nobel prize for the Photoelectric Effect but not for his work on general relativity. The photoelectric effect does however have huge implications for modern physics and provides insight into the principles of Quantum Mechanics.

What is observed experimentally when electromagnetic radiation strikes the surface of a metal is that the electrons are emitted. This occurrence is what is known as the Photoelectric Effect. The energy of these electrons can be determined by adding a electrical potential. As one increments the voltage higher and higher one will find a voltage where no more electrons will reach the other plate. From this voltage one can find the kinetic energy of the electrons. Classical theory leads to the belief that both the intensity of the light and the frequency of the light will increase the energy of the ejected electrons. However, the kinetic energy of the electrons emitted does not depend on the intensity of the beam but rather it increases linearly with the frequency of the light. Increasing the intensity of the beam will increase the number of electrons emitted but not their energies. Depending on the type of the metal, there will be a minimum frequency for which and electron will be emitted. The following equation summarizes this:

$hv=\phi +E_{k_{max}}\,$

where phi is the binding energy of the metal, h is planck's constant, and v is the frequency.

All of this is significant because in classical theory there should be no threshold for electron emissions.

Side Note: Red light emits no electrons regardless of intensity.

Particle-Wave Duality and de Broglie Wavelength

• de Broglie Hypothesis
• everything in nature has both wave and particle nature
• ${\lambda }=h/p$
• Davidsson Germer experiment
• Thompson Reid experiment

Heisenberg's Uncertainty Principle

• Measurement always disturbs object
• Deterministric view of nature is fundamentally flawed

You cannot know both position and momentum simultaneously

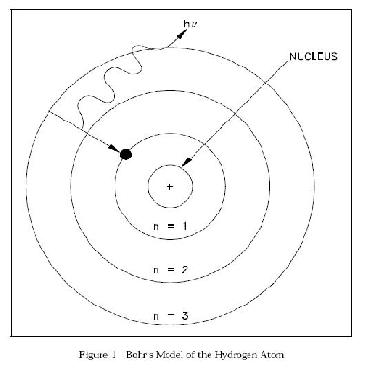
Atomic Spectra

The atomic line spectra is the phenomena where photons are absorbed and emitted by atoms at characteristic frequencies. Classically, according to the Lorentz theory, an electron in orbit around a nucleus is accelerated, causing it to emit electromagnetic radiation. This must be accompanied by a decrease in kinetic energy of the electron, causing it to spiral into the nucleus and become unstable. This is not what happens however as the electrons can only orbit around the nucleus in specified orbits with defined energies. The energies at each level are given by:

$E=-hR/n^{2}$

I need to shrink this

• Spectra Lines and Atomic Spectra
• Plum Pudding model of atom
• Rutherford's scattering experiment
• Planetary model contradicts experiments

1. Stability of matter
2. Atomic Spectra lines

• Bohr's Model 1913 basic hypothesis

1. Electrons in atoms follow classical physics and Rutherford's atomic model
2. blah blah
3. blah blah

• Bohr's theory applied to hydrogen like atoms

1. yadda yadda

• Line Spectra Rydberg's

Atomic Shell Structure

• quantum numbers (principal, orbital, magnetic, spin)
• Selectron rule in quantum transitions N = +- 1
• Electron configurations
• Pauli expclusion principle
• Periodic table

Schrödinger Equation

For 1 particle in 3D, the time-independent Schrödinger Equation is as follows

$i\hbar {\frac {\partial }{\partial t}}\Psi (\mathbf {r} ,\,t)=-{\frac {\hbar ^{2}}{2m}}\nabla ^{2}\Psi (\mathbf {r} ,\,t)+V(\mathbf {r} )\Psi (\mathbf {r} ,\,t)$

where

• $\mathbf {r} =(x,y,z)$ is the particle's position in three-dimensional space,
• $\Psi (\mathbf {r} ,t)$ is the wavefunction, which is the amplitude for the particle to have a given position r at any given time t.
• $m$ is the mass of the particle.
• $V(\mathbf {r} )$ is the potential energy of the particle at each position r.

This describes many things but not waves at a very high energy

Sources

• Description of Modern Physics - Real Wikipedia & Syllabus written by Ingo Wiedenhover