User:DimitriosLazarou

Feynman diagrams

Complete calculations of Green's functions is a rather formidable task. Even the basic imaginary time evolution operator itself is an infinite series to all orders in the interaction $V({\vec {r}},\tau )$. One can simply get lost in the dozens of integrals; his physical intuition also doesn't get things any better. Feynman diagrams are both an exact mathematical representation of perturbation theory in infinite order and a powerful pictorial method showing in a unique way the physical content of a given expression.

The introduction of Feynman diagrams to Solid State Physics came naturally in order to give an alternate, and more easy to use, way to apply perturbative concepts beyond lowest orders. Given the so-called Feynman rules, a process can be illustrated in a much more transparent way. This alternate formulation is completely equivalent with the algebraic one. The correspondence with Quantum Field Theory, (QED, QCD and more) where the diagrams were first introduced, is more than obvious. Julian Schwinger once said rather bitterly that "Feynman brought quantum field theory to the masses". This will become apparent at the end.

Simple examples

The quantity of interest is a Green's function. The language used more often in QFT is the propagator. More precisely we are interested in the n-th order corrections of it. Of course even from the 2nd order things become slightly complicated as we will see. Let's begin with no interaction at all, just the calculation of the probability of the propagation of a particle from position x to position y, or in a more accurate language the quantity $\left\langle \phi (x)\phi (y)\right\rangle$ which as we know is represented by a Green's function, i.e. $G(x-y)$. In the language of Feynman diagrams it means that we draw an internal line at x and an external line at y.

The internal line is represented by a leg (line) having an arrow that moves to a vertex (a solid dot) and the external line by a leg moving from a vertex .

In order to construct the simplest diagram of all, we connect the two vertices in the only possible way, a line:

and by simplifying things we keep only the in between part

which is nothing else but $G(x-y)$.

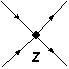
1st order correction Let's try now a more complicated vertex, we will demonstrate the 1st order correction to Green's function. Let's suppose that we have 2 fields again $\phi (x),\phi (y)$, one in position x and another in y. . Hence we have 2 lines as before. But now the vertex we put in between (position z) is this one, we call it vertex $\phi ^{4}(z)$: