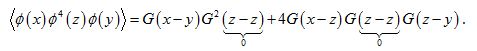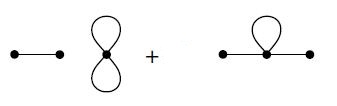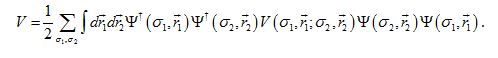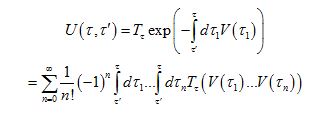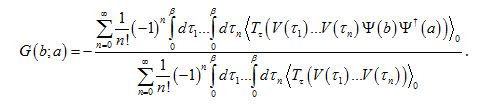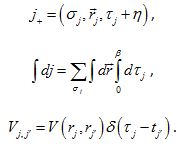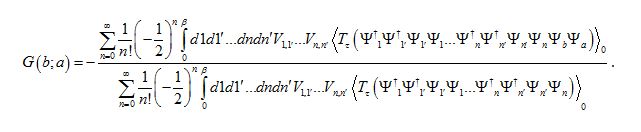User:DimitriosLazarou

Feynman diagrams

Complete calculations of Green's functions is a rather formidable task. Even the basic imaginary time evolution operator itself is an infinite series to all orders in the interaction $V({\vec {r}},\tau )$. One can simply get lost in the dozens of integrals; his physical intuition also doesn't get things any better. Feynman diagrams are both an exact mathematical representation of perturbation theory in infinite order and a powerful pictorial method showing in a unique way the physical content of a given expression.

The introduction of Feynman diagrams to Solid State Physics came naturally in order to give an alternate, and more easy to use, way to apply perturbative concepts beyond lowest orders. Given the so-called Feynman rules, a process can be illustrated in a much more transparent way. This alternate formulation is completely equivalent with the algebraic one. The correspondence with Quantum Field Theory, (QED, QCD and more) where the diagrams were first introduced, is more than obvious. Julian Schwinger once said rather bitterly that "Feynman brought quantum field theory to the masses". This will become apparent at the end.

Simple examples

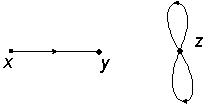
The quantity of interest is a Green's function. The language used more often in QFT is the propagator. More precisely we are interested in the n-th order corrections of it. Of course even from the 2nd order things become slightly complicated as we will see. Let's begin with no interaction at all, just the calculation of the probability of the propagation of a particle from position x to position y, or in a more accurate language the quantity $\left\langle \phi (x)\phi (y)\right\rangle$ which as we know is represented by a Green's function, i.e. $G(x-y)$. In the language of Feynman diagrams it means that we draw an internal line at x and an external line at y.

The internal line is represented by a leg (line) having an arrow that moves to a vertex (a solid dot) and the external line by a leg moving from a vertex .

In order to construct the simplest diagram of all, we connect the two vertices in the only possible way, a line:

and by simplifying things we keep only the in between part

which is nothing else but $G(x-y)$.

1st order correction Let's try now a more complicated vertex, we will demonstrate the 1st order correction to Green's function. Let's suppose that we have 2 fields again $\phi (x),\phi (y)$, one in position x and another in y. . Hence we have 2 lines as before. But now the vertex we put in between (position z) is this one, we call it vertex $\phi ^{4}(z)$:

Now we should connect the two lines, external and internal, with the above vertex in all possible ways. One way is just to connect x with y and ignore the vertex. Of course we take into account the vertex lines and we draw the appropriate (directional in this case) loops between them:

(If there are no arrows, our loops also have no arrows, we will show it next, in 2nd order correction).

Now the other possibility is to connect x with z and z with y. In our case it can be done in 2 ways as far as the internal line is concerned because there are 2 ingoing lines in the vertex, and in other 2 ways for the external lines, total of 4 ways. The lines from the vertex that remain unconnected, form a loop as before. Let's illustrate this, (having in mind that the topology of the following two diagrams is the same though and that in the future they will be represented as one diagram):

Therefore the three above diagrams represent the total average $\left\langle \phi (x)\phi ^{4}(z)\phi (y)\right\rangle$ which now can be analyzed in 2 terms that contain the Green's functions, first term for the first unconnected diagram and the 2nd term for the other two connected diagrams:

The simplification in terms of Feynman diagrams is obvious. This is the 1st order correction to the Green function.

2nd order correction

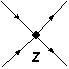
The 2nd order correction contains 2 vertices at z, z' positions, with no arrows now:

We want to compute $\left\langle \phi (x)\phi ^{4}(z')\phi (y)\right\rangle$. Let's just write the final result, no arrows this time to make it easier. The numbers in front of the diagrams are called combinatorial factors and they are obviously positive integers; they represent the total ways that this particular topology can be realized:

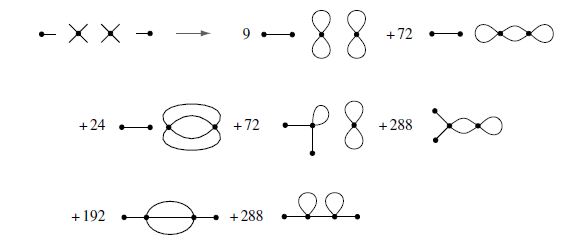
The problem of determining the combinatorics remains highly non-trivial. For example in the next correction, (3rd), the number of terms are (14-1)!!=135135. We will address it later. We will highlight some general remarks of Feynman diagrams and then we will attempt to calculate a more interesting case of interacting fermions.

General remarks

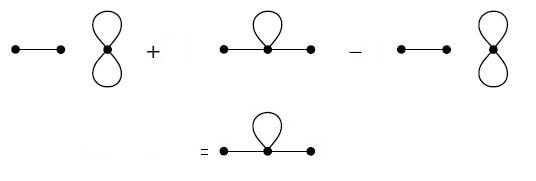
- Feynman diagrams represent the perturbative expansion. In order to be able to compute it we need the rules that relate the objects which consist the graph to analytical expressions. These are called Feynman rules, we will develop them later, for now we can just say that (i) we attach coordinates to all field lines and all of the vertices (ii) lines are the representation of Green's functions (propagators or correlation functions) (iii) multiply the graph by the factor ${\frac {g^{n}}{n!}}$ (iv) integrate over all the internal coordinates, special care in loops (v) switching from coordinate space to momentum representation of the various quantities is possible and sometimes makes things easier. (vi) To obtain the Green's function we need to add the contributions emanating from the expansion of the denominator of the functional average. We will explicitly show it later. - The diagrams can be stretched, mirror inverted, twisting, rotating and otherwise deformed; only their topology matters. Also loops that are linked only to external or internal lines, can be removed in order to end up with a so-called "amputated" diagram. A loop linked only to an external / internal line represents a slight perturbation in our incoming particle beam, it's arbitrary. - We can attach a loop order to any diagram which is the number of inequivalent loops formed by segments of lines that correspond to Green functions. - When an interaction vertex does not connect to any of the external vertices, we call it vacuum graph. This graph does not correspond to the correction of Green's function in that order. Formally one can see that by expanding the denominator of the correspondent Green's function and by observing the exact cancellation of the vacuum graph's contribution (linked cluster theorem). For example in the 1st order perturbation theory, excluding the arrows for a second, we got these two different topologies:

But the first one is a vacuum graph. Therefore the graph that will eventually contribute to our calculation is only the second one. Pictorially:

Hence in the 2nd order of perturbation theory the diagrams that contribute are the following:

Fermions with pair interactions - The Feynman diagrams treatment Let's illustrate how we can incorporate FD into a real-life example, system of fermions with pair interactions. The unperturbed Hamiltonian is while the interaction term is written as

Obviously $H=H_{0}+V$. will derive the Feynman rules for the diagrammatic expansion in orders of V of the following Green's function For simplicity we will write . The $\Psi$ fields are given in Heisenberg representation, we will convert them to Interaction representation. By subscript "0" we mean averages with respect to Failed to parse (Conversion error. Server ("https://wikimedia.org/api/rest_") reported: "Cannot get mml. TeX parse error: Double exponent: use braces to clarify"): {\displaystyle e^{-}^{\beta }^{H}_{0})} . We write for G:

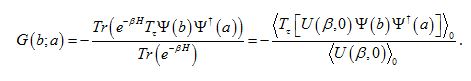
We will need the help of the following expansion, also known from QFT, it's the time-ordering operator expansion:

Now we insert this expansion into the G(b;a) relation above, adjusted to our needs. We get:

As always in these cases we are cautious about the ordering of creation and annihilation operators. The equation for V above forces us to keep creation operators to the left. This restriction can be realized formally in another more clear way. We add in infinitesimal time $\eta =0^{+}$ in the $\Psi ^{+}$ fields to make sure that the time ordering is right, i.e. the time coordinate in $\Psi ^{+}$ fields is always greater than the time coordinate in $\Psi$ fields. of (b;a) we had before denoting all the indices, we switch to "j+" notation as:

Hence the tau-integrals of V in the above large expression can be written in the light of this new notation as

Armed with this machinery we can go back to the big expression for G(b;a) and rewrite it:

The averages are with respect to $H_{0}$ only. Actually the average of the products of field operators in the numerator is the bare (2n+1)-particle Green's function $G_{0}^{2n+1}(b,1,1,...,n';a,1,1,...,n')$ times a factor of $(-1)^{2n+1}=-1$. Denominator is $G_{0}^{2n}(1,1,...,n';1,1,...,n')$ times $(-1)^{2n}=1$, hence the overall minus sign is taking care with another one. Using Wick's theorem for the above Green's functions we write G(b;a) in terms of the determinants containing the bare single particle Green's function $G^{0}(l,j)$. Let's do it:

Derivation of Feynman rules based on G(b;a):

We will derive the Feynman rules for the denominator and then for the numerator. We will see that a cancellation will take place exactly as before in the trivial examples. Here we will see it happening in detail. (i) lines: this is equal to the bare single particle Green's function $G^{0}(j_{2},j_{1})$. As always in this case $\tau _{1}$ is equal to $\tau _{1}+\eta$