6th Week: The Early Universe and Big Bang Nucleosynthesis
The Big Bang Cosmology
Cosmological principle
The cosmological principle, which is the more general version of the Copernican principle, states that on large spatial scales, the Universe is homogeneous and isotropic. This means that there is no special point in the Universe. Homogeneity of the universe means that the universe has the same property at any regions from point to point. Isotropy of the universe means that the universe looks the same from all directions. We know that at small scales the universe is not homogenous and not isotropic otherwise any structures e.g. galaxies, stars, planets and humans would not even exist. However provided that we consider the universe on average on large scales, it looks approximately homogenous and isotropic. The observed cosmological scales are therefore approximately Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle 300} to Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle 500} Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle Mpc} in which the cosmological principle works.
The expanding Universe
Before 1915, it was believed that the cosmos was static and infinite. But the infinite Universe (Newtonian Universe) was ruled out soon due to the Olbers paradox, that states for such a Universe the dark night should not exist. The Einsteins theory of gravitation suggested that the Universe is no more static. But however in order to get a static Universe solution Einstein added a so called cosmological constant, Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \Lambda} which later he called it his greatest blunder. In 1922 Friedmann solved the Einsteins equations for isotropic and homogeneous universe and found that the Universe is either expanding or collapsing. This was experimentally discovered (the galaxies of the universe all receed from each other) by Hubble in 1928, that the Universe is expanding and the expansion law is the following
- Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \vec{v} = H_0 \vec{R} \ , }
where Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle H_0} is the so called Hubble constant, which at present believed to be
- Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle H_0 = 70.1 \pm 1.3 \quad (km/s)/Mpc \ . }
The Hubble "constant" is not a constant, but is actually a time varying quantity. Defining a scale factor, Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle a} ,
- Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \vec{R} = a \vec{x} \ , }
where Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \vec{x}} is the comoving coordinate, one can find the folowing relation
- Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle H_{o} = \frac{\dot{a}(t_{o})}{a(t_{o})} \ . }
Friedmann equations
From the large-scale distribution of galaxies and the near-uniformity of the CMB temperature, we have good evidence that the universe is nearly homogeneous and isotropic. Under this assumption, the space-time metric can be written in the form
- Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle ds^2 = -c^2dt^2 + a^2(t)\left[\frac{\mathrm{d}r^2}{1-k r^2} + r^2 \mathrm{d}\mathbf{\Omega}^2\right] \ , }
where
- Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \mathrm{d}\mathbf{\Omega}^2 = \mathrm{d}\theta^2 + \sin^2 \theta \, \mathrm{d}\phi^2 \ . }
There are two independent Friedmann equations for modeling a homogeneous, isotropic universe. They are:
- Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle H^2 = \left(\frac{\dot{a}}{a}\right)^2 = \frac{8 \pi G}{3} \rho - \frac{kc^2}{a^2} + \frac{\Lambda c^2}{3}}
which is derived from the 00 component of Einstein's field equations, and
- Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \dot{H} + H^2 = \frac{\ddot{a}}{a} = -\frac{4 \pi G}{3}\left(\rho+\frac{3p}{c^2}\right) + \frac{\Lambda c^2}{3}}
which is derived from the trace of Einstein's field equations.
Using the first equation, the second equation can be re-expressed as
- Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \dot{\rho} = -3 H \left(\rho + \frac{p}{c^2}\right) \ , }
which eliminates Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \Lambda \!} and expresses the conservation of mass-energy.
Now remembering to consider that our geometry is in Euclidean space with time as dimension also.
If the scale factor Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle a} is taken to be 1 at the present time, Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle k} describes the spatial curvature when Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle a = 1} (i.e. today).
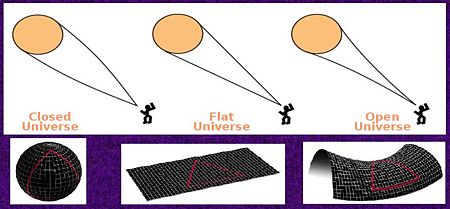
We arise with three possible scenarios.
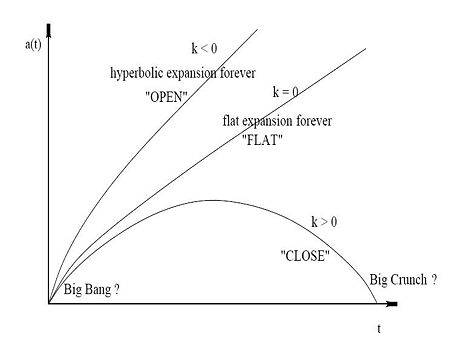
If Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle k} is positive, then the Universe is geometrically viewed as a hyperspherical, or also called as a closed Universe.
In the closed Universe the expansion stops at some point and then recollapses to a Big Crunch.
If Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle k} is zero, then the universe is flat.
The flat Universe expands forever.
If Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle k} is negative, then the universe is hyperbolic in geometry and also called an open Universe.
The open Universe expands with acceleration and the end result is known as the Big Chill.
for arbitrary k and a mixture of vacuum energy and relativistic and non-relativistic matter, we have
Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \;\; \rho = \frac{3H_{o}^{2}}{8\pi G}\left [\Omega _{\Lambda } +\Omega _{M }(\frac{a_{o}}{a})^{3}+\Omega _{R }(\frac{a_{o}}{a})^{4}\right ]}
Solution of the Friedmann equations
For simplicity, from now on we work in the units of Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle c=1} . Then the fluid equation is just
- Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \dot{\rho} = -3 H \left(\rho + p\right) \ . }
The equation of state generally can be written as
- Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle p = w \rho \ , }
where Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle w=0} for cosmological dust, Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle w=1/3} for radiation, Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle w=-1} for cosmological constant or vacuum energy. The general solution is then
- Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \rho =\rho_0 \left(\frac{a}{a_0}\right)^{-3(1+w)} \ . }
And the solution to the Friedmann equation gives
- Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle a =a_0 \left(\frac{t}{t_0}\right)^{2/[3(1+w)]} \ . }
Evolution of density with time
Putting the las two equations together we obtain
- Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \rho(t) = \rho_0 \left(\frac{t}{t_0}\right)^{-2} \ , }
where the subscript Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle 0} denotes as the quantity for today. Thus, the density evolves the same regardless of the type of the fluid. Further substitution defines the Hubble constant in terms of time
- Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle H(t) = \left(\frac{2}{3(1+w)}\right)\frac{1}{t} \ . }
Relying on the observation of Hubble constant and using the equation of state parameter one can find the approximate age of the Universe. For a dust-filled Universe this time is equal to
- Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle t_0 = \frac{2}{3H_0} \approx 9.2 \times 10^9 \quad years \ . }
Primordial Nucleosynthesis
Big bang nucleosynthesis begins with the individual baryons: protons and neutrons. The neutrons are unstable as free particles but due to the shortness of time during the nucleosynthesis era of the big bang, their abundance is only slightly reduced by this decay.
The reactions on the free nucleons, the proton and the neutron, are most important in the time of beta equilibrium and, after freeze out, when the weak reactions eventually end reacting slower than the cooling time and the free neutrons decay.
At Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle 0.3} Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle Mev} , nuclei become favoured over free nucleons. Apparently here, the production rate is larger than the photon destruction rate. All free neutrons left (number of neutrons after freeze out minus number of neutrons destroyed due to free neutron decay) get bound up to Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle He-4}
- Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle T_{freeze}= 1.5 \times 10^{4}K (\Omega _{b}h^{2}) \ . }
Most of the mass in the universe is not in the form of ordinary baryonic matter (electrons & atomic nuclei).
Puzzlement of the Big Bang Theory
Here we assume simply that the universe went through an early period of exponential expansion with no care how the hell it happened for the moment. This is done to go about a review of three classic cosmological problems.
Flatness
The flatness problem was first mentioned by Robert Dicke in 1969. It is a cosmological fine-turning problem, and the most accepted solution is cosmic inflation, along with the monopole problem and the horizon problem.
The problem is that that the density of the unverse The flatness problem is a cosmological fine-tuning problem within the Big Bang model; i.e., the observation that the current density of the universe is very close to its critical value at which space is perfectly flat. Since the total energy density of the universe departs rapidly from the critical value over cosmic time,[1] the early universe must have had a density even closer to the critical density (see below), leading cosmologists to question how the density of the early universe came to be fine-tuned to this 'special' value.
The problem was first mentioned by Robert Dicke in 1969. The most commonly-accepted solution among cosmologists is cosmic inflation; along with the monopole problem and the horizon problem, the flatness problem is one of the three primary motivations for inflationary theory.
Horizons
The puzzlement we come across here arises from the much high degree of isotropy of the Cosmic Microwave Background.
the proper horizon size at the time of last scattering Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle t_{L} }
Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle d_{H}(t_{L})\equiv a(t_{L})\int_{t_{\ast}}^{t_{L}}\frac{dt}{a(t)}}
we set the beginning of the era of inflation at Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle t_{\ast}}
Monopoles
Inflation as the way out of the problems
A sort of solution to the cosmological problem.