PHZ3400-09 Emergence in Condensed Matter

“ The whole is more than the sum of its parts ” -Aristotle

Group 3 is the best

Emergence Artist's view of Emergence Classical Macroscopic Center of Mass Phases Solid Liquid Gas Plasma Quantum Solidity Macroscopic Quantum

Emergence is the formation of complex patterns from simpler parts and basic interactions.

Emergence can be more professionally defined as: "the arising of novel and coherent structures, patterns and properties during the process of self-organization in complex systems" according to Professor Jeffrey Goldstein in the School of Business at Adelphi University.

The following points concern the scope of matter that we're studying, from the smallest quantum mechanics to the largest macroscopic molecules.

Granularity

Do we have to watch every atom?

Are we concerned about the macroscopic or the microscopic? This is a question of granularity.

The answer depends on the context of the problem.

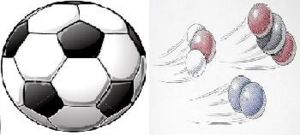
If one is looking at the motion of a soccer ball as a whole as if it were a particle, then the answer is no. In this case, we are comprising our system of large components, mainly the soccer ball. The particles of air inside the ball move randomly, and for the most part have no impact on the movement of the ball as a whole, so there is no point in keeping track of every particle in the ball. This is an example of coarse-graining, where only large particles comprise the system of interest. In condensed matter physics, scientists often begin with a microscopic system and use coarse graining to achieve a description of a macroscopic system.

If one wanted to know the air pressure inside the ball, knowing properties of the individual particles is more convenient and the answer is mostly yes. This is an example of fine-grained system.

When deciding on using a coarse-grained or fine-grained system, one should decide if the property desired is macroscopic or microscopic.

Macroscopic Bodies

A macroscopic body is made up of many microscopic bodies usually on the order of at least one mole. The amount of particles in one mole is given by Avogadro's number, on the order of ${\mathit {10^{23}}}$ particles. Were every particle to be watched, there would be something on the order of $e^{10^{23}}$ states to solve for, a significantly high number capable of being too much for even the best computers.

Center of Mass

Rather than tracking every atom, we simplify things by using only the center of mass to draw a complete picture about the object. This allows for a few degrees of freedom.

The center of mass $\mathbf {R}$ of a system of particles is defined as the average of their positions, $\mathbf {r} _{i}$, weighted by their masses, $m_{i}$:

$\mathbf {R} ={\sum m_{i}\mathbf {r} _{i} \over \sum m_{i}}.$

For a continuous distribution with mass density $\rho (\mathbf {r} )$ and total mass $M$, the sum becomes an integral:

$\mathbf {R} ={\frac {1}{M}}\int \mathbf {r} \;dm={\frac {1}{M}}\int \rho (\mathbf {r} )\,\mathbf {r} \ dV={\frac {\int \rho (\mathbf {r} )\,\mathbf {r} \ dV}{\int \rho (\mathbf {r} )\ dV}}.$

If an object has uniform density then its center of mass is the same as the centroid of its shape. Center of mass is an example of a collective co-ordinate in which all of the particles are treated as one.

Phases of Matter

Snow Flake

Matter is observed macroscopically as a set of collective and observable coordinates, as opposed to a collection of wiggling atoms. On Earth, matter comes in 3 phases depending on temperature: solid, liquid, and gas. There is one more phase that can only be found on the sun: plasma. But we're not concerned about that here.

Phase Transitions

There are 2 types of phase transitions:

• 1st order phase transitions, characterized by a change in heat or pressure
• 2nd order phase transitions, characterized by spontaneous symmetry breaking

Carbon Phase Diagram. Different types of solid carbon are formed at different pressures

First order phase transition

Car is like a diamond

This is a phase transition where order parameters (such as temperature and energy) jump discontinuously at the phase transition.

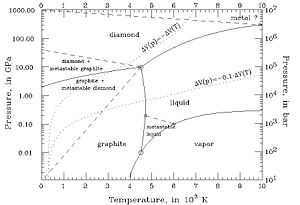
Water can come in solid (like the snowflake shown), liquid, and gaseous forms. In water, the change in temperature governs these transitions. In compounds such as carbon chains, the change in pressure creates different types of the same state, "allotropes". For carbon as a solid, this includes graphite, coal, and diamonds. The Equation of States is:

$PV=nk_{B}T\;$

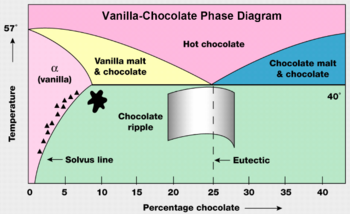
Another method of phase shifting can be seen in the vanilla-chocolate phase diagram. As one can see the percent of chocolate in the vanilla-chocolate mixture also can cause a phase shift. Though this example appears simplistic, compounds do exhibit property changes as the proportions of the constituent elements change.

Phase diagram used in a chocolate factory

Second order phase transition

These phase transitions are continuous and quantum. When a system starts in one phase and must transition to another, the system goes from a state where it is in equilibrium to a mixture of states. This mixture of states is the symmetry breaking. In order for symmetry breaking to occur, a system must be sufficiently large. This means that the number of particles $n\rightarrow \infty$. Once this condition has been met, the system has reached the thermodynamic limit and spontaneous symmetry breaking can occur. In physics, the zero-point energy is the lowest possible energy that a quantum mechanical physical system may have. This is the energy of the ground state.

Magnetization

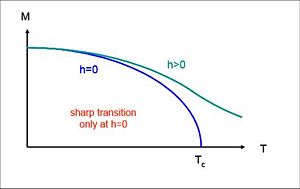
Spin susceptibility $\chi ={\frac {dM(t)}{dH}}$ and near the critical temperature $\chi ={\frac {H}{T-\theta }}$ at $T>>T_{c}$ and $\chi =(T_{R}-T)^{-\gamma }$ at $T<T_{c}$

The critical exponent $\gamma =1.3$ is the sum for large classes of materials.

Universality is the observation that there are properties for a large class of systems that are independent of the dynamical details of the system. Systems display universality in a scaling limit, when a large number of interacting parts come together. The modern meaning of the term was introduced by Leo Kadanoff in the 1960s, but a simpler version of the concept was already implicit in the Van-Der-Waals equation and in the earlier Landau theory of phase transitions, which did not incorporate scaling correctly. This simpler version, mean field theory, is useful in simplifying problems.

Importance of Quantum Mechanics

The cause of these phase changes is an interesting question. Can these phase changes be found by classical or quantum mechanical means?

For example, why don't we suffer the fate of Rumpelstiltskin (drop through the floor)?

This is because objects are solid.

But is solidity a quantum or classical phenomena?

Since solidity is macroscopically observed it should be a macroscopic property, especially since solids contain many particles. But classical physics gives no reason for objects to be solid other than molecular vibrations from the temperature of the solid. So ideally one could manipulate the temperature and affect the solidity of the floor. Except for the extreme case where the temperature is brought to the level where the floor dissociates, temperature does not affect solidity.

However, quantum mechanics can solve this problem. Heisenberg's uncertainty principle forces the electrons to have discrete values. This also means that the orbitals are incompressible unless the electrons can orbit in the same energy level (that is, possess non distinct quantum numbers). The Pauli Exclusion principle and Fermi-Dirac statistics show that electrons cannot orbit the same energy level, because electrons are fermions and therefore cannot occupy the same quantum state simultaneously. Without quantum mechanics, we wouldn't be able to explain the stability of atomic matter, why doesn't everything spiral to the center of a nucleus, or why you cannot make an electron's orbit smaller.

So despite solidity being macroscopically observed, it is quantum mechanics that keeps solids from passing through each other.

Phase diagram of high temperature superconductors: chemically doped ceramics (magnetic insulators) to introduce mobile charge carriers.